U.S. Dept. of Commerce / NOAA / OAR / PMEL / Publications
Buoyancy-driven plumes in rotating, stratified cross flows: Plume dependence on
rotation, turbulent mixing, and cross-flow strength
J. W. Lavelle
National Oceanic and Atmospheric Administration/Pacific Marine Environmental
Laboratory, Seattle, Washington
Journal of Geophysical Research, 102(C2), 3405-3420 (1997)
Not subject to U.S. copyright. Published in 1997 by the American Geophysical Union.
Results
Six model experiments are reported here. Discussion begins with a reference
case followed by contrasting results of perturbed cases (Table 1). Perturbed
cases refer to those at zero rotation rate ( = 0), null shear-dependent turbulent mixing intensity (CS
= 0, (6)), reduced horizontal background mixing (AHMIN = 10
= 0), null shear-dependent turbulent mixing intensity (CS
= 0, (6)), reduced horizontal background mixing (AHMIN = 10 m
m s
s , (4)),
and higher U
, (4)),
and higher U of 3 and 6 cm
s
of 3 and 6 cm
s . The three velocity cases to be compared
have R = wMAX /U
. The three velocity cases to be compared
have R = wMAX /U values of 6.6, 2.8, and 1.0, where wMAX is the computed maximum
upward velocity in the plume stem and U
values of 6.6, 2.8, and 1.0, where wMAX is the computed maximum
upward velocity in the plume stem and U is background flow velocity. Buoyancy dominates plume development for larger
R and cross flow dominates for smaller R. The parameter R
is often used to classify situations of jets entering cross flows, in which
case wMAX represents jet exit velocity. Sykes
et al. [1986] ran numerical experiments for jets that spanned the ratio
2-8. Laboratory experiments of Ernst
et al. [1994] on buoyant jets in cross flows showed plume bifurcation
over the range R = 2-6.
is background flow velocity. Buoyancy dominates plume development for larger
R and cross flow dominates for smaller R. The parameter R
is often used to classify situations of jets entering cross flows, in which
case wMAX represents jet exit velocity. Sykes
et al. [1986] ran numerical experiments for jets that spanned the ratio
2-8. Laboratory experiments of Ernst
et al. [1994] on buoyant jets in cross flows showed plume bifurcation
over the range R = 2-6.
Calculated fields in three dimensions and in time include  ,
p
,
p ,
,  ,
S, and AI. This paper focuses primarily on temperature
and velocity/vorticity fields near temporal equilibrium as a way to describe
differences caused by rotation, turbulence, and cross-flow speed.
,
S, and AI. This paper focuses primarily on temperature
and velocity/vorticity fields near temporal equilibrium as a way to describe
differences caused by rotation, turbulence, and cross-flow speed.
Reference Case
Figures 2 and 3
present cross sections through the reference experiment plume at 24 hours past
plume startup. With the given advection speed (U = 1.5 cm s
= 1.5 cm s ), the advection distance over
that time period is 3 times the distance (425 m) between source and outflow
boundary. By 24 hours, the plume is in equilibrium; heat flux through the outflow
boundary is equal to the heat input at the source. Even as early as 8 hours,
corresponding to an advection distance just past the outflow boundary, the plume
is in equilibrium around the source and near equilibrium at outflow, with outflow
heat flux already 80% of vent heat input.
), the advection distance over
that time period is 3 times the distance (425 m) between source and outflow
boundary. By 24 hours, the plume is in equilibrium; heat flux through the outflow
boundary is equal to the heat input at the source. Even as early as 8 hours,
corresponding to an advection distance just past the outflow boundary, the plume
is in equilibrium around the source and near equilibrium at outflow, with outflow
heat flux already 80% of vent heat input.
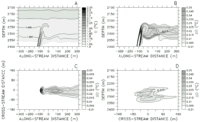
Figure 2. Plume distributions for the reference case (experiment 21,
Table 1) at t = 24 hours. The cross-stream direction is y and
the along-stream direction is x. (a)  on the plane y = 0; (b)
on the plane y = 0; (b) 
 on the plane y = 0; (c)
on the plane y = 0; (c) 
 on the plane z = 2280 m; (d)
on the plane z = 2280 m; (d) 
 on the plane x = 320 m. All contours are in degrees Celsius.
on the plane x = 320 m. All contours are in degrees Celsius.
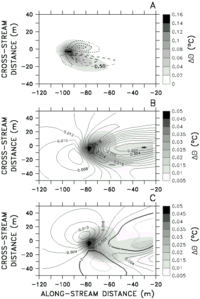
Figure 3. Velocity, 
 ,
and relative vorticity patterns for the reference case (experiment 21, Table
1) at t = 24 hours. (a)
,
and relative vorticity patterns for the reference case (experiment 21, Table
1) at t = 24 hours. (a) 
 (shaded) and
(shaded) and  z nondimensionalized
by U
z nondimensionalized
by U /D (contoured)
at z = 2350 m (z/D = 5); (b)
/D (contoured)
at z = 2350 m (z/D = 5); (b) 
 (shaded) and u velocity (contoured) at z = 2280 (z/D =
12); (c)
(shaded) and u velocity (contoured) at z = 2280 (z/D =
12); (c) 
 (shaded) and v velocity (contoured) at z = 2280 (z/D
= 12). Velocity is in meters per second.
(shaded) and v velocity (contoured) at z = 2280 (z/D
= 12). Velocity is in meters per second.
Contours of  (Figure
2a) show the effect of hydrothermal heat release on the surrounding environment.
Isotherms are drawn down into the source region. The inverted J-shaped isotherm
and a plume stem bent ~13° with respect to the vertical evidence the effects
of background flow. The well-defined stem has lateral
(Figure
2a) show the effect of hydrothermal heat release on the surrounding environment.
Isotherms are drawn down into the source region. The inverted J-shaped isotherm
and a plume stem bent ~13° with respect to the vertical evidence the effects
of background flow. The well-defined stem has lateral  gradients as large as 6.8 × 10
gradients as large as 6.8 × 10 °C m
°C m .
Relatively steep gradients such as these are not numerically easy to preserve;
their occurrence in these calculations results from the use of the upstream
corrected advection scheme of Smolarkiewicz
and Clark [1986]. Downstream internal waves above the height of the
convection column with wavelength of ~140-170 m are also evident in Figure
2a. These are associated with internal waves in u and w velocities
with amplitudes of ~0.2 and 0.6 cm s
.
Relatively steep gradients such as these are not numerically easy to preserve;
their occurrence in these calculations results from the use of the upstream
corrected advection scheme of Smolarkiewicz
and Clark [1986]. Downstream internal waves above the height of the
convection column with wavelength of ~140-170 m are also evident in Figure
2a. These are associated with internal waves in u and w velocities
with amplitudes of ~0.2 and 0.6 cm s , respectively.
Lees waves in the atmosphere resulting from convective motion have been modeled
by Hauf
and Clark [1989] and are used to good advantage by glider pilots [Kuettner
et al., 1987]. A possible explanation for their occurrence is that a
convection column can act, in part, like a hill, forcing environmental flow
over and around, but further numerical experimentation is required to be unequivocal
about the cause in this setting.
, respectively.
Lees waves in the atmosphere resulting from convective motion have been modeled
by Hauf
and Clark [1989] and are used to good advantage by glider pilots [Kuettner
et al., 1987]. A possible explanation for their occurrence is that a
convection column can act, in part, like a hill, forcing environmental flow
over and around, but further numerical experimentation is required to be unequivocal
about the cause in this setting.
The  anomaly,
anomaly, 
 =
=  -
-  BKG,
contoured in units of 0.005°C (Figure 2b), better
shows the maximum rise of the plume to be 180 m. For the given combination of
U
BKG,
contoured in units of 0.005°C (Figure 2b), better
shows the maximum rise of the plume to be 180 m. For the given combination of
U and B
and B ,
the plume overshoots the neutral density level. Yet flow is strong enough, with
the given source buoyancy and stratification, that the overshoot is small, and
strong enough that no limb of the plume appears upstream of the source. For
fixed B
,
the plume overshoots the neutral density level. Yet flow is strong enough, with
the given source buoyancy and stratification, that the overshoot is small, and
strong enough that no limb of the plume appears upstream of the source. For
fixed B , an upstream limb
can be expected as U
, an upstream limb
can be expected as U is reduced
[e.g.,
Ernst et al., 1994], and full plume symmetry about the then-vertical
convection axis must occur when U
is reduced
[e.g.,
Ernst et al., 1994], and full plume symmetry about the then-vertical
convection axis must occur when U = 0 [e.g.,
Lavelle, 1995]. In all cases reported here, U
= 0 [e.g.,
Lavelle, 1995]. In all cases reported here, U is sufficiently large (
is sufficiently large ( 1.5 cm/s) that neither
upstream nor significant cross-stem plume growth occurs. Flows in and around
the stem and plume cap region, which result from the superposition of background
and convective flows, are also much different than flows expected in and around
convective plumes rising into a quiescent background environment [e.g.,
Lavelle and Baker, 1994].
1.5 cm/s) that neither
upstream nor significant cross-stem plume growth occurs. Flows in and around
the stem and plume cap region, which result from the superposition of background
and convective flows, are also much different than flows expected in and around
convective plumes rising into a quiescent background environment [e.g.,
Lavelle and Baker, 1994].
Vertical velocities in the stem reach maxima of 0.1 m s in this example. The plume overshoots the equilibrium level and causes a slight
positive density anomaly
in this example. The plume overshoots the equilibrium level and causes a slight
positive density anomaly 
 in the region above the stem. Just downstream of the positive
in the region above the stem. Just downstream of the positive 
 region, flow has a downward directed component with vertical velocities of as
much as 0.03 m s
region, flow has a downward directed component with vertical velocities of as
much as 0.03 m s . The local maximum of
. The local maximum of 
 centered near x = 50 m (Figure 2b) is
at the terminal end of this downward directed flow. Note that
centered near x = 50 m (Figure 2b) is
at the terminal end of this downward directed flow. Note that 
 > 0.02 °C extends only several hundred meters downstream because of lateral
dispersion. Since resolving
> 0.02 °C extends only several hundred meters downstream because of lateral
dispersion. Since resolving 

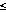 0.01 °C in field data is difficult, a single
hydrothermal source of the given size in this stratification environment ought
to be difficult to detect thermally beyond several hundred meters from the vent
source.
0.01 °C in field data is difficult, a single
hydrothermal source of the given size in this stratification environment ought
to be difficult to detect thermally beyond several hundred meters from the vent
source.
A planar view of the same plume at 120 m above the bottom (z = 2280
m, Figure 2c) shows the small aspect ratio of
the plume for the given cross-flow strength. At the outflow boundary, the plume
(i.e., the 0.005°C isopleth) at this depth is ~100 m wide. Growth of the plume
in the flow transverse direction is limited by the downstream transport of plume
material. The local maximum centered at x = 75 m (Figure
2c) is the same local maximum evident in Figure
2b, the consequence of initial plume overshoot with subsequent downward
advection. Computations without rotation show planar distributions with perfect
symmetry about the y = 0 axis. The slight asymmetry of the pattern of
Figure 2c is thus caused by rotation, a topic
to be taken up more fully later. No undesirable outflow boundary layer is apparent
in Figures 2a-2c, evidence that supports Johansson's
[1993] prescription for boundary conditions.
At the outflow boundary (x = 320 m), the equilibrium plume shows a maximum
core 
 of 0.015°C
(Figure 2d). The plume (i.e., 0.005°C isopleth)
has maximum width of ~200 m, about half the width of the computational domain
(320 m). Cyclic boundary conditions in the y direction allow transport
through the side walls of the calculational region, but little
of 0.015°C
(Figure 2d). The plume (i.e., 0.005°C isopleth)
has maximum width of ~200 m, about half the width of the computational domain
(320 m). Cyclic boundary conditions in the y direction allow transport
through the side walls of the calculational region, but little  -distribution
contamination by adjoining cyclic domains is apparent. A wider computational
domain width would be necessitated if smaller U
-distribution
contamination by adjoining cyclic domains is apparent. A wider computational
domain width would be necessitated if smaller U were used. A test experiment was performed to examine the change in results
due to quadrupling the domain width. In that case the y-direction resolution
was coarsened to 10 m, but all other aspects of the calculations were left intact.
Results were not significantly different from those shown here. In consideration
of computational costs, most experiments were run with the 320-m domain width.
were used. A test experiment was performed to examine the change in results
due to quadrupling the domain width. In that case the y-direction resolution
was coarsened to 10 m, but all other aspects of the calculations were left intact.
Results were not significantly different from those shown here. In consideration
of computational costs, most experiments were run with the 320-m domain width.
Circulation in the region of the plume stem and above is considerably different
from that predicted for point source convection in otherwise quiescent environments
[e.g.,
Lavelle and Baker, 1994]. In the high 
 -gradient
region near the source,
-gradient
region near the source, 
 contours (shaded, Figure 3a) are kidney- or horseshoe-shaped,
as found numerically, for example, of plumes in nonrotating environments by
Sykes
et al. [1986]. The same authors cite numerous laboratory observations
of the same effect. While much of the upstream fluid enters the stem, there
is also some spatial acceleration of flow around the stem. Downstream of the
stem there is a u-velocity minimum and beyond that is a reconvergence
of the stem-separated flow. The flow patterns result in a counterrotating
contours (shaded, Figure 3a) are kidney- or horseshoe-shaped,
as found numerically, for example, of plumes in nonrotating environments by
Sykes
et al. [1986]. The same authors cite numerous laboratory observations
of the same effect. While much of the upstream fluid enters the stem, there
is also some spatial acceleration of flow around the stem. Downstream of the
stem there is a u-velocity minimum and beyond that is a reconvergence
of the stem-separated flow. The flow patterns result in a counterrotating  z
couplet (contours, Figure 3a) with a
z
couplet (contours, Figure 3a) with a  z
maximum on the right (referenced to the downstream direction) and a minimum
on the left, at the downstream end of the
z
maximum on the right (referenced to the downstream direction) and a minimum
on the left, at the downstream end of the 
 anomalies. Such a counterrotating
anomalies. Such a counterrotating 
 couplet at the downstream edge of a jet entering cross flow was noted by Turner
[1960] and observed by Moussa
et al. [1977], for example. Besides the asymmetry caused by rotation
(Figure 3a), the
couplet at the downstream edge of a jet entering cross flow was noted by Turner
[1960] and observed by Moussa
et al. [1977], for example. Besides the asymmetry caused by rotation
(Figure 3a), the  z
pair is also much like the one found numerically by Sykes
et al. [1986] for jets in nonrotating, unstratified cross flows. Not
shown is the perturbation pressure (p') distribution, which has two local
minima of comparable size located asymmetrically about y = 0 and slightly
downstream of the
z
pair is also much like the one found numerically by Sykes
et al. [1986] for jets in nonrotating, unstratified cross flows. Not
shown is the perturbation pressure (p') distribution, which has two local
minima of comparable size located asymmetrically about y = 0 and slightly
downstream of the 
 isopleth tips.
isopleth tips.
The kidney-shaped pattern of 
 extends from the seafloor to the levels of neutral buoyancy. At z = 2280
m, the pattern of
extends from the seafloor to the levels of neutral buoyancy. At z = 2280
m, the pattern of 
 in the stem region (shaded, Figures 3b and 3c)
also has two lobes, the right lobe being larger. Asymmetry about y =
0 is again the consequence of Coriolis forces. Superimposed on the
in the stem region (shaded, Figures 3b and 3c)
also has two lobes, the right lobe being larger. Asymmetry about y =
0 is again the consequence of Coriolis forces. Superimposed on the 
 distributions are isopleths of u (Figure 3b)
and v (Figure 3c). With background flow
at 0.015 m s
distributions are isopleths of u (Figure 3b)
and v (Figure 3c). With background flow
at 0.015 m s , Figure
3b shows a region of reduced u ahead of the convection column and
a region of near-zero u some 20 m downstream. The v distribution
(Figure 3c) shows maxima to both side of the
column, but much higher v in the direction of positive y. Downstream,
the signs of the two v lobes reverse to allow the reconvergence of the
flow that was deflected to either side of the column. Though Figure
3 bears evidence that the Coriolis force does affect plume structure, the
simple anticyclonic flow for the upper plume predicted when convection occurs
in a quiescent background environment [Lavelle
and Baker, 1994] no longer occurs. Additional differences in plume structure
with and without rotation are discussed in the following section.
, Figure
3b shows a region of reduced u ahead of the convection column and
a region of near-zero u some 20 m downstream. The v distribution
(Figure 3c) shows maxima to both side of the
column, but much higher v in the direction of positive y. Downstream,
the signs of the two v lobes reverse to allow the reconvergence of the
flow that was deflected to either side of the column. Though Figure
3 bears evidence that the Coriolis force does affect plume structure, the
simple anticyclonic flow for the upper plume predicted when convection occurs
in a quiescent background environment [Lavelle
and Baker, 1994] no longer occurs. Additional differences in plume structure
with and without rotation are discussed in the following section.
The Nonrotating Case
A nonrotational case was next examined. To isolate direct effects of rotation,
the u profile of Figure 1a was taken as
the along-stream background current, and cross-stream v was taken to
be zero in experiment 22. Using (11), a 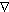 P
P profile consistent with those velocity profiles but unique to the nonrotating
case was determined. The resulting
profile consistent with those velocity profiles but unique to the nonrotating
case was determined. The resulting 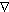 P
P was used to force ambient cross flow (Table 1). Using the
was used to force ambient cross flow (Table 1). Using the 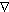 P
P of experiment 21 when
of experiment 21 when 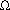 = 0 would have resulted
in a uBKG profile with a much thicker boundary layer.
= 0 would have resulted
in a uBKG profile with a much thicker boundary layer.
The primary difference in 
 caused by rotation is the absence of distributional symmetry about y
= 0; rise height, overshoot, and magnitudes are otherwise similar in a general
sense. As expected, u and v too are symmetrical about y
= 0 in experiment 22 (Figures 4a and 4b) but
not in experiment 21 (Figures 4c and 4d). To
allow easier comparison of these results with those of Sykes
et al. [1986], velocities and distances in Figure
4 have been non-dimensionalized by U
caused by rotation is the absence of distributional symmetry about y
= 0; rise height, overshoot, and magnitudes are otherwise similar in a general
sense. As expected, u and v too are symmetrical about y
= 0 in experiment 22 (Figures 4a and 4b) but
not in experiment 21 (Figures 4c and 4d). To
allow easier comparison of these results with those of Sykes
et al. [1986], velocities and distances in Figure
4 have been non-dimensionalized by U and D.
and D.
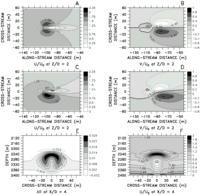
Figure 4. Comparison of rotating (experiment 21) and nonrotating (experiment
22) cases. Velocity u nondimensionalized by U on the plane z = 2380 m (z/D = 2) when
on the plane z = 2380 m (z/D = 2) when 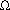 = 0 (Figure 4a) and when
= 0 (Figure 4a) and when 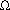
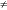 0 (Figure 4c).
Velocity v nondimensionalized by U
0 (Figure 4c).
Velocity v nondimensionalized by U on the plane z = 2380 m (z/D = 2) when
on the plane z = 2380 m (z/D = 2) when 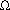 = 0 (Figure 4b) and when
= 0 (Figure 4b) and when 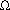
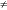 0 (Figure 4d).
For
0 (Figure 4d).
For 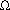 = 0,
= 0, 
 on the plane x = -60 m (x/D = 4) (Figure
4e) and nondimensional u on the plane x = -60 m (x/D
= 4) (Figure 4f).
on the plane x = -60 m (x/D = 4) (Figure
4e) and nondimensional u on the plane x = -60 m (x/D
= 4) (Figure 4f).
Figures 4a (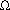 = 0) and 4c (
= 0) and 4c (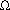
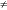 0) show u in the vicinity of the
source at a vertical distance of z/D = 2, where z is distance
from the seafloor. When
0) show u in the vicinity of the
source at a vertical distance of z/D = 2, where z is distance
from the seafloor. When 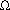 = 0, u is nearly
doubled (U/U
= 0, u is nearly
doubled (U/U = 1.9)
on both sides of the rising plume as background flow, in part, sweeps around
the ascending fluid column. The bulk of the upstream flow is undeflected; after
entering the column, u momentum is displaced vertically, with the result
that little of the u momentum entering upstream is found downstream at
z levels where the stem is well-defined. The u velocity immediately
downstream of the stem is just greater than zero, although flow of small size
(u < 0) occurs in the boundary layer (z/D < 2) and at some
sites above z/D = 10. Though the possibility of downstream reverse flow
(u < 0) must depend on boundary layer thickness and strength of upward
convection, the general result, that nearly all entrainment into the stem occurs
from the upstream side of plumes under similar forcing conditions, is likely
not to be significantly altered.
= 1.9)
on both sides of the rising plume as background flow, in part, sweeps around
the ascending fluid column. The bulk of the upstream flow is undeflected; after
entering the column, u momentum is displaced vertically, with the result
that little of the u momentum entering upstream is found downstream at
z levels where the stem is well-defined. The u velocity immediately
downstream of the stem is just greater than zero, although flow of small size
(u < 0) occurs in the boundary layer (z/D < 2) and at some
sites above z/D = 10. Though the possibility of downstream reverse flow
(u < 0) must depend on boundary layer thickness and strength of upward
convection, the general result, that nearly all entrainment into the stem occurs
from the upstream side of plumes under similar forcing conditions, is likely
not to be significantly altered.
In the rotating case (Figure 4c), reverse flow
(u < 0) occurs on the left side of the convection column (y
> 0), while larger along-stream flow (u/U = 2.4) occurs on the right (y < 0). This asymmetry helps shape the
= 2.4) occurs on the right (y < 0). This asymmetry helps shape the

 distribution
of, for example, Figure 3a. The region of flow
affected is small. Consequently, field observations of u enhancement
near a heat source may prove difficult, if only because of the small size of
the region involved.
distribution
of, for example, Figure 3a. The region of flow
affected is small. Consequently, field observations of u enhancement
near a heat source may prove difficult, if only because of the small size of
the region involved.
Magnitude of the transverse velocity, |v| , reaches ~0.6U in both cases (Figures 4b and 4d). For
in both cases (Figures 4b and 4d). For 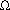 = 0, maximum |v| occurs nearly twice as far downstream as |
= 0, maximum |v| occurs nearly twice as far downstream as |
 |
maxima, which occur at the downstream edge of the stem. The v convergence
(Figure 4b) results in u values again
having magnitudes ~U
|
maxima, which occur at the downstream edge of the stem. The v convergence
(Figure 4b) results in u values again
having magnitudes ~U within
a distance of 5D downstream of x = 0. The effect of
within
a distance of 5D downstream of x = 0. The effect of 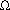
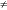 0 on v (Figure
4d) is to skew distributions across the plane of symmetry (y = 0)
so maximum |v| occurs slightly farther downstream than does maximum |-v|.
Magnitudes of v at this z level are little changed by rotation.
0 on v (Figure
4d) is to skew distributions across the plane of symmetry (y = 0)
so maximum |v| occurs slightly farther downstream than does maximum |-v|.
Magnitudes of v at this z level are little changed by rotation.
For cases when R = 4 and R = 8, Sykes
et al. [1986] provide distributions of a passive scalar and u
on the cross section x/D = 4. While their study of jets involved
neither background stratification nor a boundary layer, their results show horseshoe-shaped
patterns for the scalar (as in Figure 4e) and
u distributions with a low velocity core underlying a higher velocity
high region (as in Figure 4f). Results here show
larger vertical gradients above the 
 core (Figure 4e) and maximum u velocities
the distribution of which drapes less over the sides of the lower u core
(Figure 4f) than it does in the results of Sykes
et al. [1986]. Those differences undoubtedly reflect the presence of
background stratification. In none of the panels of Figure
4 is the full domain of computation shown.
core (Figure 4e) and maximum u velocities
the distribution of which drapes less over the sides of the lower u core
(Figure 4f) than it does in the results of Sykes
et al. [1986]. Those differences undoubtedly reflect the presence of
background stratification. In none of the panels of Figure
4 is the full domain of computation shown.
Relative vorticity distributions allow a comparison to the results of Sykes
et al. [1986] as well. Nondimensionalized stream-wise vorticity 
 in the source region at z/D = 2 appears as a counterrotating couplet
(Figure 5a); even during plume development (t
< 1 hour) the couplet at this level has the indicated strength and shape.
Low p
in the source region at z/D = 2 appears as a counterrotating couplet
(Figure 5a); even during plume development (t
< 1 hour) the couplet at this level has the indicated strength and shape.
Low p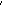 is found just downstream of extremal
is found just downstream of extremal
 x sites. If
x sites. If 
 contours were superimposed,
contours were superimposed,  x
extrema would be seen to be just downstream of the
x
extrema would be seen to be just downstream of the 
 center. When
center. When 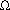
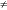 0, the axis separating the two counterrotating vortices of the couplet is oriented
clockwise of the x axis, but magnitudes are comparable to those of the
0, the axis separating the two counterrotating vortices of the couplet is oriented
clockwise of the x axis, but magnitudes are comparable to those of the
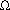 = 0 case. Sykes
et al. [1986] found distributions of
= 0 case. Sykes
et al. [1986] found distributions of 
 that are similar in both length scale and intensity at this height. The similarity
is not surprising, in that at z/D = 2 the background environment
is well mixed (Figures 1a-1b) and by this time
(t = 8.3 hours) conditions in the stem have long ago reached equilibrium.
The pattern (Figure 5a) is primarily the consequence
of the
that are similar in both length scale and intensity at this height. The similarity
is not surprising, in that at z/D = 2 the background environment
is well mixed (Figures 1a-1b) and by this time
(t = 8.3 hours) conditions in the stem have long ago reached equilibrium.
The pattern (Figure 5a) is primarily the consequence
of the  w/
w/ y
contribution to
y
contribution to  x and, for
fixed x, error function-like distributions of w in the y
direction across the plume stem; the other term contributing to
x and, for
fixed x, error function-like distributions of w in the y
direction across the plume stem; the other term contributing to  x,
x,
 v/
v/ z,
is less than 10% the size of
z,
is less than 10% the size of  w/
w/ y.
y.
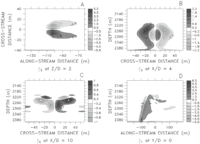
Figure 5. Relative vorticity, nondimensionalized by U /D,
about the x axis,
/D,
about the x axis, 
 ,
when
,
when  = 0 on the planes (a) z = 2380
(z/D = 2), (b) x = -60 m (x/D = 4), and (c)
x = 0 (x/D = 10). (d) Relative vorticity, nondimensionalized
by U
= 0 on the planes (a) z = 2380
(z/D = 2), (b) x = -60 m (x/D = 4), and (c)
x = 0 (x/D = 10). (d) Relative vorticity, nondimensionalized
by U /D, about the
y axis,
/D, about the
y axis, 
 ,
on the plane y = 0.
,
on the plane y = 0.
Downstream, the distribution of  x
grows in complexity. For example, on the plane x/D = 4,
x
grows in complexity. For example, on the plane x/D = 4, 
 has two pairs of counterrotating cells (Figure 5b).
The underlying velocity field is like that measured by Fearn
and Weston [1974] for a jet entering a cross flow. It is the w
distribution, similar to Figure 4e, once differentiated
(i.e.,
has two pairs of counterrotating cells (Figure 5b).
The underlying velocity field is like that measured by Fearn
and Weston [1974] for a jet entering a cross flow. It is the w
distribution, similar to Figure 4e, once differentiated
(i.e.,  w/
w/ y),
much more than the distribution of
y),
much more than the distribution of  v/
v/ x,
that determines the form of
x,
that determines the form of 
 .
At this x location,
.
At this x location,  x
again resembles that found by Sykes
et al. [1986]. At x/D = 10, where negative w at
the level of neutral buoyancy is a response to the initial plume overshoot (Figure
3a),
x
again resembles that found by Sykes
et al. [1986]. At x/D = 10, where negative w at
the level of neutral buoyancy is a response to the initial plume overshoot (Figure
3a), 
 is preponderantly negative on the right side and positive on the left (Figure
5c). No similar result could be expected for jets in homogenous flow because
then no vertical overshoot is possible. Stratification also broadens and flattens
the
is preponderantly negative on the right side and positive on the left (Figure
5c). No similar result could be expected for jets in homogenous flow because
then no vertical overshoot is possible. Stratification also broadens and flattens
the  x distribution at this
distance.
x distribution at this
distance.
In experiment 22 ( = 0), initially only
= 0), initially only 
 is nonzero, and then only in the boundary layer, because background shear is
at first unidirectional. Both
is nonzero, and then only in the boundary layer, because background shear is
at first unidirectional. Both 
 and
and 
 quickly develop, however, and
quickly develop, however, and 
 is substantially changed as convectively forced flow develops. On the plane
y = 0, for example, singlet
is substantially changed as convectively forced flow develops. On the plane
y = 0, for example, singlet 
 becomes a couplet extending much higher into the water column (Figure
5d) with extremal values (6.5 units) that dwarf original magnitudes (-0.65
units). The distribution of
becomes a couplet extending much higher into the water column (Figure
5d) with extremal values (6.5 units) that dwarf original magnitudes (-0.65
units). The distribution of 
 in Figure 5d is also determined by the distribution
of w across the stem region:
in Figure 5d is also determined by the distribution
of w across the stem region:  w/
w/ x,
the significant factor determining
x,
the significant factor determining 
 ,
is positive entering the stem on the upstream side and negative exiting the
stem downstream. Sykes
et al. [1986] and Klemp
[1987], among others, have analyzed the growth of vorticity components as
convection occurs, so a full discussion of that time development is unnecessary
to repeat here. Klemp
[1987] shows that when cross-stream vorticity is present, it is tilted and
drawn up by buoyancy driven flow during thunderstorm development to initiate
a
,
is positive entering the stem on the upstream side and negative exiting the
stem downstream. Sykes
et al. [1986] and Klemp
[1987], among others, have analyzed the growth of vorticity components as
convection occurs, so a full discussion of that time development is unnecessary
to repeat here. Klemp
[1987] shows that when cross-stream vorticity is present, it is tilted and
drawn up by buoyancy driven flow during thunderstorm development to initiate
a  z couplet (e.g., Figure
3a). Schlesinger
[1980] suggests that no initial shear is needed for all three
z couplet (e.g., Figure
3a). Schlesinger
[1980] suggests that no initial shear is needed for all three  components to develop, but tilting by advection is a primary means of growth
for downstream
components to develop, but tilting by advection is a primary means of growth
for downstream  components during storm development.
components during storm development.
Dominance of  x and
x and 
 by one of the horizontal derivatives of w points to the certainty of
development of both relative vorticity components starting at the time buoyancy
is first generated because vertical velocity is created by buoyancy from startup.
For example, without cross flow the distribution of w in the budding
stem would be Gaussian in both lateral directions, and the first derivative
of w would lead to counterrotating vorticity pairs in both x and
y directions, i.e.,
by one of the horizontal derivatives of w points to the certainty of
development of both relative vorticity components starting at the time buoyancy
is first generated because vertical velocity is created by buoyancy from startup.
For example, without cross flow the distribution of w in the budding
stem would be Gaussian in both lateral directions, and the first derivative
of w would lead to counterrotating vorticity pairs in both x and
y directions, i.e.,  x and
x and
 y. Clearly no cross-stream
or stream-wise vorticity is needed initially when buoyancy forcing is present
to generate
y. Clearly no cross-stream
or stream-wise vorticity is needed initially when buoyancy forcing is present
to generate  x and
x and 
 .
Just as clearly does
.
Just as clearly does  z production
begin at the same time: as the convection column first deflects a fraction of
the background flow to both sides, flow that subsequently converges downstream,
distributions of u and v (Figures 4a-4d)
are created that once differentiated lead to nonzero
z production
begin at the same time: as the convection column first deflects a fraction of
the background flow to both sides, flow that subsequently converges downstream,
distributions of u and v (Figures 4a-4d)
are created that once differentiated lead to nonzero  z.
Production of all components of
z.
Production of all components of  must occur
at any location where buoyancy has begun to disturb background flow.
must occur
at any location where buoyancy has begun to disturb background flow.
Sensitivity to Mixing Strength
To gauge sensitivity of results to subgrid-scale mixing, two additional experiments
were performed. In the first, dependence of mixing on shear (equation (6)) was
eliminated by setting CS = 0, so only constant mixing coefficients
controlled turbulent diffusion (experiment 23). Those background mixing coefficients
had relatively small values AZMIN (10 m2 s
m2 s ) and AHMIN
(10
) and AHMIN
(10 m2 s
m2 s ),
as indicated earlier. In another experiment (experiment 26), CS
was left at 0.2 so that AI (equation (6)) would be a significant
factor in mixing in the stem region, where shears are larger, but the value
of AHMIN that controls lateral mixing outside that region
was reduced by a factor of 10. In this case AHMIN is smaller
(10
),
as indicated earlier. In another experiment (experiment 26), CS
was left at 0.2 so that AI (equation (6)) would be a significant
factor in mixing in the stem region, where shears are larger, but the value
of AHMIN that controls lateral mixing outside that region
was reduced by a factor of 10. In this case AHMIN is smaller
(10 m
m s
s )
than even AZMAX (equation (12)). In reference experiment 21,
AI in the plume stem was typically 10-20 × 10
)
than even AZMAX (equation (12)). In reference experiment 21,
AI in the plume stem was typically 10-20 × 10 m2 s
m2 s . With a small value in experiment
26, AHMIN had little influence on mixing in the stem region
and much reduced influence beyond. Effects of stirring by nonlinear advection
beyond the convection region are thus highlighted in experiment 26.
. With a small value in experiment
26, AHMIN had little influence on mixing in the stem region
and much reduced influence beyond. Effects of stirring by nonlinear advection
beyond the convection region are thus highlighted in experiment 26.
Results at t = 8.3 hours for the three cases are shown in Figure
6. In both panels, 
 isopleths are provided as solid lines (experiment 23), dotted lines (experiment
26), or shaded regions (experiment 21). Both experiments with reduced turbulent
mixing show
isopleths are provided as solid lines (experiment 23), dotted lines (experiment
26), or shaded regions (experiment 21). Both experiments with reduced turbulent
mixing show  anomalies with greater spatial
variability. Three plumes along y = 0 (Figure 6a)
show that there is no substantial difference in the equilibrium level of the
plumes. Using height of maximum
anomalies with greater spatial
variability. Three plumes along y = 0 (Figure 6a)
show that there is no substantial difference in the equilibrium level of the
plumes. Using height of maximum 
 averaged over each section in the downstream interval 200 < x <
320 m as indicator, average rise heights were 136, 125, and 122 m for experiments
21, 23, and 26, respectively (Table 1). Thus reduced lateral mixing leads to
only slightly smaller rise heights. On the other hand, when time development
of the plumes is examined, the starting pulse of anomalous
averaged over each section in the downstream interval 200 < x <
320 m as indicator, average rise heights were 136, 125, and 122 m for experiments
21, 23, and 26, respectively (Table 1). Thus reduced lateral mixing leads to
only slightly smaller rise heights. On the other hand, when time development
of the plumes is examined, the starting pulse of anomalous  water rose to a height greater by 27 m in the case of smallest stem viscosity
(experiment 23) compared to the case of largest viscosity (experiment 21). Earlier
work by Lavelle
and Baker [1994] for plumes without cross flow had shown higher rise
heights with less stem mixing. Present results agree only for the initial interval
of rise to the level of neutral buoyancy, but not in the longer term. The explanation
must lie in differences in entrainment when cross flow is present. In the cross
flow case, background flow is forced into the plume stem region on the upstream
side, while without cross flow, entrainment is caused by convection alone and
occurs omnidirectionally.
water rose to a height greater by 27 m in the case of smallest stem viscosity
(experiment 23) compared to the case of largest viscosity (experiment 21). Earlier
work by Lavelle
and Baker [1994] for plumes without cross flow had shown higher rise
heights with less stem mixing. Present results agree only for the initial interval
of rise to the level of neutral buoyancy, but not in the longer term. The explanation
must lie in differences in entrainment when cross flow is present. In the cross
flow case, background flow is forced into the plume stem region on the upstream
side, while without cross flow, entrainment is caused by convection alone and
occurs omnidirectionally.
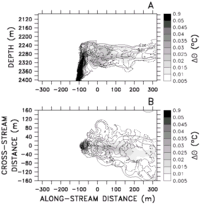
Figure 6. 
 for experiments that differ only in the subgrid-scale parameterization (Table
1). Results are represented by dotted contours for experiment 26 (AHMIN
= 10 cm
for experiments that differ only in the subgrid-scale parameterization (Table
1). Results are represented by dotted contours for experiment 26 (AHMIN
= 10 cm s
s ),
solid contours for experiment 23 (CS = 0), and shaded contours
for experiment 21, the reference case. (a) Cross sections for y = 0,
(b) cross sections for z = 2280 m. All contours are in degrees Celsius
at 8.3 hours.
),
solid contours for experiment 23 (CS = 0), and shaded contours
for experiment 21, the reference case. (a) Cross sections for y = 0,
(b) cross sections for z = 2280 m. All contours are in degrees Celsius
at 8.3 hours.
When viewed on the horizontal plane z = 2280 m, plumes of experiments
23 and 26 show larger lateral downstream spread than in the reference experiment
(Figure 6b). Counterintuitively, smaller mixing
coefficients either locally in the stem (experiment 23) or globally (experiment
26) cause greater lateral dispersion. Using the 0.005°C 
 isopleth to designate a plume edge, widths averaged over 200 < x <
320 m for the three experiments were 90, 188, and 155 m, respectively. In experiment
26, that same
isopleth to designate a plume edge, widths averaged over 200 < x <
320 m for the three experiments were 90, 188, and 155 m, respectively. In experiment
26, that same 
 edge shows signs of wispiness, as if Helmholtz shear instabilities were occurring.
edge shows signs of wispiness, as if Helmholtz shear instabilities were occurring.
Statistics of  z within the
downstream plume (
z within the
downstream plume (
 > 0.005°C, x > 100 m ) show experiment 26 having larger relative
vorticity. In experiment 26,
> 0.005°C, x > 100 m ) show experiment 26 having larger relative
vorticity. In experiment 26,  z
was more patchy downstream of the source. Mean values of |
z
was more patchy downstream of the source. Mean values of | z|
for the three experiments were 1.4 ×, 1.2 ×, and 2.9 × 10
z|
for the three experiments were 1.4 ×, 1.2 ×, and 2.9 × 10 s
s , while the standard deviation of |
, while the standard deviation of |
 |
for experiment 26 is twice as large as for the other experiments. This suggests
that resolved stirring rather than unresolved mixing is more significant as
a dispersion process in experiment 26 than in the others. Thus reduced turbulent
mixing in the far field, i.e., smaller AHMIN, allows stronger
small-scale stirring, which in turn leads to more widespread plume dispersal.
|
for experiment 26 is twice as large as for the other experiments. This suggests
that resolved stirring rather than unresolved mixing is more significant as
a dispersion process in experiment 26 than in the others. Thus reduced turbulent
mixing in the far field, i.e., smaller AHMIN, allows stronger
small-scale stirring, which in turn leads to more widespread plume dispersal.
Differences in velocities also are apparent with changes in turbulent mixing
intensity. In the stem, w maxima are smallest with full mixing (experiment
21) and largest when CS = 0 (experiment 23). Downstream negative
w are 40% larger in experiment 23 than experiment 21. Times series show
that differences are much more than just changes in magnitude. Animations of

 fields show
source heat, while steadily discharged at the seafloor, being pulsed to higher
levels when mixing is small (experiments 23 and 26) but not when mixing is higher
(experiment 21). Time series sampled at a site 120 m above and 27.5 m downstream
of the source (Figure 7) show, in comparison,
that
fields show
source heat, while steadily discharged at the seafloor, being pulsed to higher
levels when mixing is small (experiments 23 and 26) but not when mixing is higher
(experiment 21). Time series sampled at a site 120 m above and 27.5 m downstream
of the source (Figure 7) show, in comparison,
that 
 quickly
grows to a value of 0.06°C in all three experiments as the plume front passes
but thereafter they are quite different. For largest mixing (experiment 21),
quickly
grows to a value of 0.06°C in all three experiments as the plume front passes
but thereafter they are quite different. For largest mixing (experiment 21),

 smoothly
seeks an equilibrium level. In experiments 23 and 26, on the other hand,
smoothly
seeks an equilibrium level. In experiments 23 and 26, on the other hand, 
 values oscillate with periods of ~1300 s. The w time series at this location
shows similar frequency content. Buoyancy period, based on the linear region
of the
values oscillate with periods of ~1300 s. The w time series at this location
shows similar frequency content. Buoyancy period, based on the linear region
of the  profile (Figure
1b), is 1265 s. The oscillation period from model results is only a coarse
estimate because model data were sampled only every 300 s. Since the amplitude
of
profile (Figure
1b), is 1265 s. The oscillation period from model results is only a coarse
estimate because model data were sampled only every 300 s. Since the amplitude
of 
 oscillations
is nearly 0.03°C in experiment 26 (Figure 7),
field observations of
oscillations
is nearly 0.03°C in experiment 26 (Figure 7),
field observations of  very near to hydrothermal
heat sources at intervals of 1 min or less might be able to distinguish different
mixing coefficient regimes.
very near to hydrothermal
heat sources at intervals of 1 min or less might be able to distinguish different
mixing coefficient regimes.
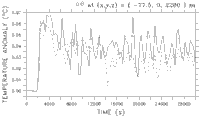
Figure 7. Time series of 
 at a fixed point in the plume stem (x/D = 2.25, y = 0,
z/D = 12). The dotted line represents the experiment of largest
subgrid-scale mixing (experiment 21), while the other two have reduced background
mixing (dashed line, experiment 26) or shear independent mixing (solid line,
experiment 23).
at a fixed point in the plume stem (x/D = 2.25, y = 0,
z/D = 12). The dotted line represents the experiment of largest
subgrid-scale mixing (experiment 21), while the other two have reduced background
mixing (dashed line, experiment 26) or shear independent mixing (solid line,
experiment 23).
Changes in Cross-Flow Strength
Effects of cross-flow strength were examined by increasing U from 1.5 cm s
from 1.5 cm s in experiment 21, to 3 and
6 cm s
in experiment 21, to 3 and
6 cm s in experiments 24 and 25, while all
other conditions were held fixed. That sequence of three experiments has R
= wMAX/Uo values of 6.6, 2.8, and 1.0, respectively,
where wMAX is maximum upward stem velocity determined empirically
from results of each experiment. This range of R is comparable to that
examined by Sykes
et al. [1986] and is approximately the range over which Ernst
et al. [1994], in laboratory experiments, found significant changes
in the character of buoyant jets in cross flows.
in experiments 24 and 25, while all
other conditions were held fixed. That sequence of three experiments has R
= wMAX/Uo values of 6.6, 2.8, and 1.0, respectively,
where wMAX is maximum upward stem velocity determined empirically
from results of each experiment. This range of R is comparable to that
examined by Sykes
et al. [1986] and is approximately the range over which Ernst
et al. [1994], in laboratory experiments, found significant changes
in the character of buoyant jets in cross flows.
Plumes bend increasingly with increasing cross-flow strength, as expected (Figures
8a and 8d); in all panels of Figure 8 the
dotted line represents the 
 = 0.005°C isotherm of experiment 21. Rise heights, hRISE,
based on the location of maximum
= 0.005°C isotherm of experiment 21. Rise heights, hRISE,
based on the location of maximum 
 in vertical sections at the outflow boundary for each of the three experiments
are 136, 97, and 77 m, respectively, the last value representing the higher
of two
in vertical sections at the outflow boundary for each of the three experiments
are 136, 97, and 77 m, respectively, the last value representing the higher
of two 
 maxima
(Figure 8f). On the basis of those three values
alone, a best fit of hRISE to U
maxima
(Figure 8f). On the basis of those three values
alone, a best fit of hRISE to U gives hRISE
gives hRISE 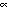 U
U -0.40.
Extensive atmospheric observations have led to the canonical form hRISE
= 2.6 [B
-0.40.
Extensive atmospheric observations have led to the canonical form hRISE
= 2.6 [B /(U
/(U N
N )]1/3
for bent-over plumes in the stratified atmosphere [e.g.,
Hanna et al., 1982], where hRISE refers to distance
between source level and the vertical midpoint of the plume downstream of the
source. Thus, for atmospheric cases, hRISE
)]1/3
for bent-over plumes in the stratified atmosphere [e.g.,
Hanna et al., 1982], where hRISE refers to distance
between source level and the vertical midpoint of the plume downstream of the
source. Thus, for atmospheric cases, hRISE 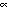 U
U -0.33. The similarity
of U
-0.33. The similarity
of U dependence for these
model results and atmospheric data is encouraging, though the paucity of model
realizations, the difficulty of defining rise height when the distribution has
more than a single maximum (Figure 8f), and the
difference in rise height definitions between this and the atmospheric case
are all causes for caution.
dependence for these
model results and atmospheric data is encouraging, though the paucity of model
realizations, the difficulty of defining rise height when the distribution has
more than a single maximum (Figure 8f), and the
difference in rise height definitions between this and the atmospheric case
are all causes for caution.
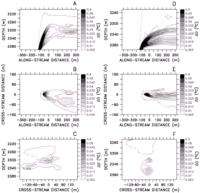
Figure 8. Contours of 
 in degrees Celsius at 8.3 hours for experiment 24 (U
in degrees Celsius at 8.3 hours for experiment 24 (U = 0.03 m s
= 0.03 m s , Table 1) at (a) y = 0,
(b) z = 2320 m, (c) x = 300 m, and for experiment 25 (U
, Table 1) at (a) y = 0,
(b) z = 2320 m, (c) x = 300 m, and for experiment 25 (U = 0.06 m s
= 0.06 m s , Table 1) at (d) y = 0,
(e) z = 2360 m, and (f) x = 300 m. The dotted contour in each
panel represents the 0.005°C isopleth for the reference case (experiment 21).
, Table 1) at (d) y = 0,
(e) z = 2360 m, and (f) x = 300 m. The dotted contour in each
panel represents the 0.005°C isopleth for the reference case (experiment 21).
Unexpectedly, plumes of experiments 24 and 25 have voids in the downstream

 distributions.
For experiment 23 (R = 2.8) this occurs just downstream of the stem,
but the branches merge again farther downstream (Figure
8a). In experiment 26 (R = 1.0), branching occurs farther from the
stem region and extends to the outflow boundary (Figure
8d). No such
distributions.
For experiment 23 (R = 2.8) this occurs just downstream of the stem,
but the branches merge again farther downstream (Figure
8a). In experiment 26 (R = 1.0), branching occurs farther from the
stem region and extends to the outflow boundary (Figure
8d). No such 
 voids were seen in experiment 21 (R = 6.6, Figure
2).
voids were seen in experiment 21 (R = 6.6, Figure
2).
In neither of the two cases is branching simple. For R = 2.8, a section
for z = 2320 m (Figure 8b) shows that
the 
 void
does not extend laterally all the way across the plume. The core region of highest
void
does not extend laterally all the way across the plume. The core region of highest
 gradients has a more exaggerated kidney shape
than in experiment 21, but only the right branch spawns material downstream
at this level; the left branch is truncated. A sequence of horizontal sections
shows the
gradients has a more exaggerated kidney shape
than in experiment 21, but only the right branch spawns material downstream
at this level; the left branch is truncated. A sequence of horizontal sections
shows the 
 void to be tubular with the principal axis of the tube skewed from the vertical.
The irregularly shaped tube cuts through the plume wall, here defined as the
void to be tubular with the principal axis of the tube skewed from the vertical.
The irregularly shaped tube cuts through the plume wall, here defined as the
 = 0.005°C isopleth, on the left-hand side
below z = 2310 m, creating the left-side void seen in Figure
8b. Above z = 2310 m, the right-side wall of the plume is interrupted.
In the x direction near the stem,
= 0.005°C isopleth, on the left-hand side
below z = 2310 m, creating the left-side void seen in Figure
8b. Above z = 2310 m, the right-side wall of the plume is interrupted.
In the x direction near the stem,  is
continuous on the right-hand side below z = 2310 m and continuous on
the left-hand side above. Beyond x = ~100 m, the plume has no voids.
At x = 300 m (Figure 8c) it is wider than
high, with two local
is
continuous on the right-hand side below z = 2310 m and continuous on
the left-hand side above. Beyond x = ~100 m, the plume has no voids.
At x = 300 m (Figure 8c) it is wider than
high, with two local 
 maxima. The rightmost maximum evidently buds from the lower right-hand limb
of the kidney-shaped region, while the upper maximum buds from the higher-rising
left-hand limb.
maxima. The rightmost maximum evidently buds from the lower right-hand limb
of the kidney-shaped region, while the upper maximum buds from the higher-rising
left-hand limb.
For the plume rising into the strongest cross flow (R = 1.0, experiment
25), the picture of a top-to-bottom bifurcation suggested by Figure
8d is also not complete. The planar view (Figure
8e) shows a plume with a strong right-hand limb and a stunted left-hand
limb at z = 2360 m. A sequence of horizontal slices shows that the left-hand
limb is favored below z = 2380 m and the right-hand limb is favored above.
The left-hand limb is attached to the seafloor and extends to x = ~200
m before disappearing; the attachment is in part caused by the location of the
source at the seafloor, but effects of the low vertical resolution of the boundary
layer by the model cannot be discounted. The right-hand limb splits vertically
but does not completely separate; the section at x = 300 m (Figure
8f) shows that the bifurcation in Figure 8d
was apparent only; the two vertically aligned maxima are connected. The plume
would be earmarked as distinctly bifurcated only if 
 = 0.005°C were too small an anomaly to be observed. Thus an observational threshold
can affect judgement as to whether a plume has bifurcated or not. This result
should also serve warning that two
= 0.005°C were too small an anomaly to be observed. Thus an observational threshold
can affect judgement as to whether a plume has bifurcated or not. This result
should also serve warning that two 
 maxima in a single vertical profile in a hydrothermal region may not mean that
two venting sources, each with different B
maxima in a single vertical profile in a hydrothermal region may not mean that
two venting sources, each with different B ,
are nearby.
,
are nearby.
Distributions of 
 within these plumes might seem peculiar if it were not for field and laboratory
observations that confirm that plumes from buoyant jets can bifurcate. Scorer
[1959] noted the occurrence of plume bifurcation in ordinary chimney plumes.
Observations of bifurcating industrial stack plumes are exemplified in the report
of Fanaki
[1975]. Volcanic plume bifurcations are summarized by Ernst
et al. [1994]. Several laboratory experiments of Wu
et al. [1988] on buoyant jets in unstratified flows led to vertical
bifurcation of the kind seen in experiment 25 (Figure
8f), though in the laboratory it was a source configuration skewed with
respect to flow direction rather than environmental rotation or shear in cross
flow that broke plume symmetry about the y axis.
within these plumes might seem peculiar if it were not for field and laboratory
observations that confirm that plumes from buoyant jets can bifurcate. Scorer
[1959] noted the occurrence of plume bifurcation in ordinary chimney plumes.
Observations of bifurcating industrial stack plumes are exemplified in the report
of Fanaki
[1975]. Volcanic plume bifurcations are summarized by Ernst
et al. [1994]. Several laboratory experiments of Wu
et al. [1988] on buoyant jets in unstratified flows led to vertical
bifurcation of the kind seen in experiment 25 (Figure
8f), though in the laboratory it was a source configuration skewed with
respect to flow direction rather than environmental rotation or shear in cross
flow that broke plume symmetry about the y axis.
Mechanisms that cause jet or plume bifurcations are not completely understood,
though observations have pinpointed some conditions under which bifurcation
is likely to occur. In cases of jets entering unstratified flows, the ratio
R has been used to classify results. Ernst
et al. [1994] saw buoyant laboratory jets that clearly bifurcated when
R fell in the range 2-6, but bifurcation was blurred or did not occur
at higher or lower R values. In model plumes addressed here, bifurcation
occurred but was not complete when R = 1.0 and 2.8, but did not occur
when R = 6.6. Ernst
et al. [1994] noted that sharp density interfaces, orientation of a
jet orifice with respect to the flow, and latent heat release all can influence
the occurrence of a bifurcation. In the case of convection during severe storms,
Klemp
and Wilhelmson [1978] showed that vertical shear of environmental winds
and downdraft caused by precipitation are important to the storm splitting and
divergence process. On the basis of results reported here, it is appropriate
to add rotation, background stratification, and boundary layer shear to the
list of possible factors affecting plume bifurcation. The large number of potential
factors involved, however, will likely make the identification of conditions
and causes leading to the bifurcation of hydrothermal plumes difficult.
Return to previous section or go to next section
PMEL Outstanding Papers
PMEL Publications Search
PMEL Homepage
 = 0), null shear-dependent turbulent mixing intensity (CS
= 0, (6)), reduced horizontal background mixing (AHMIN = 10
= 0), null shear-dependent turbulent mixing intensity (CS
= 0, (6)), reduced horizontal background mixing (AHMIN = 10 m
m s
s , (4)),
and higher U
, (4)),
and higher U of 3 and 6 cm
s
of 3 and 6 cm
s . The three velocity cases to be compared
have R = wMAX /U
. The three velocity cases to be compared
have R = wMAX /U values of 6.6, 2.8, and 1.0, where wMAX is the computed maximum
upward velocity in the plume stem and U
values of 6.6, 2.8, and 1.0, where wMAX is the computed maximum
upward velocity in the plume stem and U is background flow velocity. Buoyancy dominates plume development for larger
R and cross flow dominates for smaller R. The parameter R
is often used to classify situations of jets entering cross flows, in which
case wMAX represents jet exit velocity. Sykes
et al. [1986] ran numerical experiments for jets that spanned the ratio
2-8. Laboratory experiments of Ernst
et al. [1994] on buoyant jets in cross flows showed plume bifurcation
over the range R = 2-6.
is background flow velocity. Buoyancy dominates plume development for larger
R and cross flow dominates for smaller R. The parameter R
is often used to classify situations of jets entering cross flows, in which
case wMAX represents jet exit velocity. Sykes
et al. [1986] ran numerical experiments for jets that spanned the ratio
2-8. Laboratory experiments of Ernst
et al. [1994] on buoyant jets in cross flows showed plume bifurcation
over the range R = 2-6.  ,
,  ,
S, and AI. This paper focuses primarily on temperature
and velocity/vorticity fields near temporal equilibrium as a way to describe
differences caused by rotation, turbulence, and cross-flow speed.
,
S, and AI. This paper focuses primarily on temperature
and velocity/vorticity fields near temporal equilibrium as a way to describe
differences caused by rotation, turbulence, and cross-flow speed. 


 z nondimensionalized
by U
z nondimensionalized
by U °C m
°C m 1.5 cm/s) that neither
upstream nor significant cross-stem plume growth occurs. Flows in and around
the stem and plume cap region, which result from the superposition of background
and convective flows, are also much different than flows expected in and around
convective plumes rising into a quiescent background environment
1.5 cm/s) that neither
upstream nor significant cross-stem plume growth occurs. Flows in and around
the stem and plume cap region, which result from the superposition of background
and convective flows, are also much different than flows expected in and around
convective plumes rising into a quiescent background environment 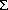 in the region above the stem. Just downstream of the positive
in the region above the stem. Just downstream of the positive 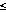 0.01 °C in field data is difficult, a single
hydrothermal source of the given size in this stratification environment ought
to be difficult to detect thermally beyond several hundred meters from the vent
source.
0.01 °C in field data is difficult, a single
hydrothermal source of the given size in this stratification environment ought
to be difficult to detect thermally beyond several hundred meters from the vent
source.  couplet at the downstream edge of a jet entering cross flow was noted by
couplet at the downstream edge of a jet entering cross flow was noted by 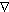 P
P profile consistent with those velocity profiles but unique to the nonrotating
case was determined. The resulting
profile consistent with those velocity profiles but unique to the nonrotating
case was determined. The resulting 
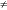 0 (
0 ( |
maxima, which occur at the downstream edge of the stem. The v convergence
(
|
maxima, which occur at the downstream edge of the stem. The v convergence
( in the source region at z/D = 2 appears as a counterrotating couplet
(
in the source region at z/D = 2 appears as a counterrotating couplet
( w/
w/
 ,
on the plane y = 0.
,
on the plane y = 0.  .
At this x location,
.
At this x location,  m2 s
m2 s

 U
U