U.S. Dept. of Commerce / NOAA / OAR / PMEL
/ Publications
Interior pycnocline flow from the Subtropical to the Equatorial
Pacific Ocean
Gregory C. Johnson and Michael J. McPhaden
NOAA, Pacific Marine Environmental Laboratory, 7600 Sand Point
Way NE, Seattle, Washington 98115-6349
Journal of Physical Oceanography, 29, 3073–3089, 1999.
This paper is not subject to U.S. copyright. Published in 1999 by the American
Meteorological Society
Abstract
Interior circulation pathways from the subtropics to the equator are markedly
different in the Northern and Southern Hemispheres of the Pacific Ocean. In
the North Pacific the pycnocline shoals and strengthens dramatically under the
intertropical convergence zone, separating the North Equatorial Current from
the North Equatorial Countercurrent. While the high potential vorticity between
these currents would intuitively seem to inhibit meridional water-property exchange
between the subtopics and the equator, transient tracer analyses and some modeling
studies have suggested an interior pathway from the subtropics to the equator
in the pycnocline of the central North Pacific. This study delineates this pathway
and estimates an upper bound for its magnitude at 5 (± 1) × 10 kg s–1. In contrast, the southern branch of the South Equatorial
Current clearly brings pycnocline water estimated at 15 (± 1) × 10
kg s–1. In contrast, the southern branch of the South Equatorial
Current clearly brings pycnocline water estimated at 15 (± 1) × 10 kg s–1 from the southern subtropics directly to the equator
in the South Pacific through an interior region of low and relatively uniform
potential vorticity. In both hemispheres, these interior pathways extend downward
as far as the lightest waters of the equatorial pycnostad. The subsurface countercurrents
flanking the pycnostad form the equatorward limbs of tropical subsurface cyclonic
gyres. These deep gyres are consistent with the absence of interior ventilation
of the equator from the subtropics below the pycnocline. Measured and derived
fields from a hydrographic climatology are presented on neutral surfaces and
in meridional-vertical sections to show that salinity, potential vorticity,
and acceleration potential are all consonant with these arguments. The vertical
extent of interior communication is also in agreement with the transient tracer
results.
kg s–1 from the southern subtropics directly to the equator
in the South Pacific through an interior region of low and relatively uniform
potential vorticity. In both hemispheres, these interior pathways extend downward
as far as the lightest waters of the equatorial pycnostad. The subsurface countercurrents
flanking the pycnostad form the equatorward limbs of tropical subsurface cyclonic
gyres. These deep gyres are consistent with the absence of interior ventilation
of the equator from the subtropics below the pycnocline. Measured and derived
fields from a hydrographic climatology are presented on neutral surfaces and
in meridional-vertical sections to show that salinity, potential vorticity,
and acceleration potential are all consonant with these arguments. The vertical
extent of interior communication is also in agreement with the transient tracer
results.
1. Introduction
Recently, it has been suggested (Gu
and Philander 1997), supported by observational analysis (Zhang
et al. 1998), that interior thermal anomalies, apparently originating
where water is subducted in the subtropics of the North Pacific (Deser
et al. 1996), may propagate to the equatorial region in the Pacific.
This propagation may occur through advection by the ocean general circulation
(Gu
and Philander 1997), or more rapidly through Rossby and Kelvin waves
(Lysne
et al. 1997). Thermal anomalies may be owing to subduction poleward
of 18°N and variations of wind-stress equatorward of this latitude (Schneider
et al. 1999). It has been further hypothesized that these anomalies
may contribute to the decadal changes observed in the structure of the equatorial
pycnocline and that this modulation of the equatorial pycnocline may in turn
be linked to a shift in the dynamics, frequency, and intensity of El Niño
(Gu
and Philander 1997). Taken together, these hypotheses are one reason
that the pathways by which and rates at which subtropical water reaches the
equatorial region is of interest in understanding and predicting seasonal-to-interannual
climate variability. Of course, describing intergyre oceanic mass and water
property exchanges, understanding their dynamics, and quantifying their magnitudes
are also important in themselves to the study of the ocean general circulation
and its role in climate.
Tritium data have been interpreted to suggest an interior pathway along which
pycnocline water ventilated in the subtropical North Pacific reaches the equator
between 145°W and 125°W (Fine
et al. 1987) in the central Pacific, crossing the intertropical convergence
zone (ITCZ) that separates westward North Equatorial Current (NEC) from the
eastward North Equatorial Countercurrent (NECC). These data suggest this northern
ventilation ranges in potential density from 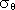 = 23 to 26.2 kg m–3 and that at
= 23 to 26.2 kg m–3 and that at 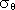 = 25.0 kg m
= 25.0 kg m the timescale for water to
reach the equator is less than 14 years.
the timescale for water to
reach the equator is less than 14 years.
Depth-integrated geostrophic mass transport in the North Pacific Ocean as inferred
from Sverdrup dynamics clearly indicates this interior pathway (McPhaden
and Fine 1988). In addition, mean surface dynamic height relative
to a pressure of 1000 dbar (Wyrtki
1975) shows a similar pattern. However, water in the ocean interior
moves along neutral surfaces. The near-surface geostrophic circulation likely
has a pattern similar to the surface dynamic height field or the depth-integrated
mass transport predicted using the wind stress curl, but these studies do not
reveal how the circulation changes with increasing density (and depth).
Modeling provides a third approach to the question of pathways for subtropical-equatorial
exchange. Ventilated thermocline dynamics (Luyten
et al. 1983), extended to the tropics in a reduced-gravity model
formulation, shows that the presence of an interior pathway for northern hemisphere
ventilation depends critically on the location of the poleward edge of the shadow
zone (McCreary
and Lu 1994; Liu
1994). A complex configuration of the model with a Pacific-like geometry
under a smoothed climatological wind field suggests that the region of high
potential vorticity beneath the ITCZ may constitute a barrier to interior subtropical-equatorial
exchange in the Northern Hemisphere (Lu
and McCreary 1995). However, reducing the model thermocline thickness
to a smaller value results in an interior pathway as one of three possibilities
(Lu
et al. 1998; Liu
and Huang 1998).
Lagrangian analysis of more complex ocean general circulation models also shows
that water subducted in the Northern Hemisphere subtropics takes one of three
pathways (Liu
et al. 1994; Gu
and Philander 1997; Liu
and Huang 1998; Rothstein
et al. 1998). Water subducted in the western North Pacific reaches
the western boundary and heads north in the Kuroshio, avoiding immediate subtropical-equatorial
exchange. Water subducted in the central North Pacific reaches the western boundary
and heads south in the Mindanao Current to join the eastward flowing Equatorial
Undercurrent (EUC), after reaching the dateline in the NECC. Water subducted
in the eastern North Pacific describes a zigzag toward the equator, initially
flowing southwestward in the NEC, then abruptly turning south to flow southeastward
in the NECC, then turning south again to flow southwestward in the northern
branch of the South Equatorial Current (SEC) before joining the EUC in the central
Pacific. In the Southern Hemisphere subtropics the three pathways for subducted
water are similar, with the significant exception that the waters subducted
farthest east flow directly northwestward toward the equator in the southern
branch of the SEC. In general, water parcels take under 16 years to reach the
equatorial region after subduction (Gu
and Philander 1997), roughly consonant with the time estimated from
the tritium measurements (Fine
et al. 1987).
A comprehensive description of the tropical Pacific Ocean circulation and its
variation with density using conventional physical oceanographic measurements
exists (Tsuchiya
1968). The work here adds to this description with discussions of
potential vorticity, vertical sections, and an emphasis on interior pathways
for subtropical-equatorial exchange, only briefly discussed in Tsuchiya
(1968). In addition, 25 times more stations are used than were available
to Tsuchiya
(1968), and the present data are all high vertical resolution CTD
data rather than the bottle data used before. Maps of depth, salinity, potential
vorticity, and acceleration potential on selected neutral surfaces are discussed
along with meridional-vertical sections of neutral density, salinity, and potential
vorticity. The density above which interior subtropical-to-equatorial exchange
ceases in both hemispheres is quantified and shown to coincide with the base
of the tropical pycnocline. This density surface outcrops in winter at the poleward
limits of the subtropical gyres. It is also the density above which the equatorial
pycnostad and the eastward flowing Tsuchiya jets, that is the North and South
Subsurface Countercurrents (NSCC and SSCC, or SCCs), are found (Tsuchiya
1975; Johnson
and Moore 1997; Rowe
et al. 2000). These jets form the equatorward limbs of basin-wide
subsurface cyclonic tropical gyres, where equatorward flow is absent except
at the western boundary. Finally, interior meridional transport estimates within
the tropical pycnocline are presented.
2. CTD data processing
For this study, CTD stations within areal bins in the tropical Pacific are
averaged to make a mean hydrographic profile for each bin. In latitude, the
bins are centered every 1° from 20°S to 20°N. In longitude, the
bins are centered every 5° from 120°E to 75°W. This anisotropy
is dictated by the data distribution, consisting mostly of closely sampled meridional
sections with wide zonal spacing, but is also appropriate to the tropical ocean
interior where zonal scales greatly exceed meridional scales. For this study
15,693 individual CTD stations between 20.5°S and 20.5°N, 120°E
and 70°W and taken from 1967 through 1998 are used. These data are all
the high-resolution profiles available from the National Oceanographic Data
Center (NODC) archives, as well as recent Pacific Marine Environmental Laboratory
(PMEL) cruise data and some other cruise data not yet available from NODC. The
average profiles are used to make maps of properties on neutral density anomaly,
 n, surfaces (Jackett
and McDougall 1997), meridional-vertical water property sections,
and mass transport estimates. Only profiles with more than two CTD casts are
used for the property maps and transport estimates and with two or more CTD
casts for the vertical sections.
n, surfaces (Jackett
and McDougall 1997), meridional-vertical water property sections,
and mass transport estimates. Only profiles with more than two CTD casts are
used for the property maps and transport estimates and with two or more CTD
casts for the vertical sections.
The CTD station data within each bin, consisting of salinity S and temperature
T as a function of pressure P are averaged as a function of  n
to create mean hydrographic profiles. Averaging S, T, and P
as functions of
n
to create mean hydrographic profiles. Averaging S, T, and P
as functions of  n is more
involved than the conventional approach of averaging S, T, and
n is more
involved than the conventional approach of averaging S, T, and
 n as functions of P,
but this technique better preserves water properties in the sharp tropical pycnocline
and the strength of the pycnocline itself because averaging is quasi-Lagrangian
in the vertical (Gouriou
and Toole 1993). First, to reduce small-scale noise, individual CTD
station S and T profiles are filtered in P with a 5-dbar
half-width Hanning filter and subsampled at 5-dbar intervals. Then
n as functions of P,
but this technique better preserves water properties in the sharp tropical pycnocline
and the strength of the pycnocline itself because averaging is quasi-Lagrangian
in the vertical (Gouriou
and Toole 1993). First, to reduce small-scale noise, individual CTD
station S and T profiles are filtered in P with a 5-dbar
half-width Hanning filter and subsampled at 5-dbar intervals. Then  n
is computed for these subsampled data and averaged as a function of P
to obtain a mean
n
is computed for these subsampled data and averaged as a function of P
to obtain a mean  n(P)
profile at 5-dbar resolution. Following this step, the individually filtered
and sampled profiles of S, T, and P are linearly interpolated
to each
n(P)
profile at 5-dbar resolution. Following this step, the individually filtered
and sampled profiles of S, T, and P are linearly interpolated
to each  n(P) profile
value to allow averaging of the individual CTD station data as a function of
n(P) profile
value to allow averaging of the individual CTD station data as a function of
 n at roughly 5-dbar resolution.
Mean profiles for S(
n at roughly 5-dbar resolution.
Mean profiles for S( n),
T(
n),
T( n), and P(
n), and P( n)
are then calculated. Finally the mean P(
n)
are then calculated. Finally the mean P( n)
profile is used to put the mean profiles of S(
n)
profile is used to put the mean profiles of S( n)
and T(
n)
and T( n) onto an
even 5-dbar grid and construct a final mean
n) onto an
even 5-dbar grid and construct a final mean  n
profile. The profile is limited to values denser than the densest mixed layer
for the CTD stations within the bin.
n
profile. The profile is limited to values denser than the densest mixed layer
for the CTD stations within the bin.
Depth and properties of the surface mixed layer, including  n,
vary over time. To construct a mixed layer for the mean profiles of S(
n,
vary over time. To construct a mixed layer for the mean profiles of S( n),
T(
n),
T( n), and
n), and  n,
the following procedure is used. First, the mixed layer P for each CTD
station is defined as the P above which
n,
the following procedure is used. First, the mixed layer P for each CTD
station is defined as the P above which  n
is less than 0.05 kg m
n
is less than 0.05 kg m denser than the
mean
denser than the
mean  n of the top 10 dbar.
Mean S and T from the surface to the mixed layer P are
calculated for each CTD station. Then these S and T values are
averaged to find mean mixed layer values for S and T. These values
are used with the mean mixed layer P to calculate a mean mixed layer
n of the top 10 dbar.
Mean S and T from the surface to the mixed layer P are
calculated for each CTD station. Then these S and T values are
averaged to find mean mixed layer values for S and T. These values
are used with the mean mixed layer P to calculate a mean mixed layer
 n. In order to avoid
n. In order to avoid  n
inversions in the mean profiles, the pressure of the mean mixed layer
n
inversions in the mean profiles, the pressure of the mean mixed layer
 n in the final mean
n in the final mean  n
profile is used to define a final mean mixed-layer P. To finish, the
mean mixed layer S, T, and
n
profile is used to define a final mean mixed-layer P. To finish, the
mean mixed layer S, T, and  n
values are substituted into the mean S(
n
values are substituted into the mean S( n),
T(
n),
T( n), and
n), and  n
profiles from the mean mixed layer P to the surface. The gap between
the mean mixed layer and the mean interior profiles is interpolated using a
shape-preserving local spline (Akima
1970).
n
profiles from the mean mixed layer P to the surface. The gap between
the mean mixed layer and the mean interior profiles is interpolated using a
shape-preserving local spline (Akima
1970).
The analysis exclusively uses the resulting 5-dbar mean hydrographic profiles.
The only further vertical smoothing performed is on the square of the buoyancy
frequency, N , which is calculated
from the mean hydrographic profiles and then smoothed with a 35-dbar median
filter before use. The median filter effectively reduces noise while preserving
the rapid transition from the surface mixed layer to the pycnocline. This quantity
is then used to calculate the planetary component of the potential vorticity,
Q = N
, which is calculated
from the mean hydrographic profiles and then smoothed with a 35-dbar median
filter before use. The median filter effectively reduces noise while preserving
the rapid transition from the surface mixed layer to the pycnocline. This quantity
is then used to calculate the planetary component of the potential vorticity,
Q = N f / g.
A few Q profiles still have some noise at depth in bins with few stations
and some of those ending in shallow water. Neutral surface maps are made by
linearly interpolating the 5-dbar values of each mean hydrographic profile to
the appropriate
f / g.
A few Q profiles still have some noise at depth in bins with few stations
and some of those ending in shallow water. Neutral surface maps are made by
linearly interpolating the 5-dbar values of each mean hydrographic profile to
the appropriate  n. These
values are then objectively mapped assuming a Gaussian covariance with correlation
length scales of 10° longitude and 2° latitude and a noise-to-signal
variance ratio of 0.25. These correlation length scales are twice the bin size,
to emphasize only those large-scale features that are well resolved by the data.
n. These
values are then objectively mapped assuming a Gaussian covariance with correlation
length scales of 10° longitude and 2° latitude and a noise-to-signal
variance ratio of 0.25. These correlation length scales are twice the bin size,
to emphasize only those large-scale features that are well resolved by the data.
3. Water property maps on neutral surfaces
Here properties on two neutral surfaces are discussed. The lighter surface,
 n = 25.0 kg m
n = 25.0 kg m ,
is chosen for proximity to the equatorial pycnocline, the EUC, and the subtropical
mode water (STMW) vertical potential vorticity minima (best seen in the meridional-vertical
sections discussed below). The denser surface,
,
is chosen for proximity to the equatorial pycnocline, the EUC, and the subtropical
mode water (STMW) vertical potential vorticity minima (best seen in the meridional-vertical
sections discussed below). The denser surface,  n
= 26.5 kg m
n
= 26.5 kg m , is chosen to lie below the
pycnocline, but within the equatorial pycnostad and the SCCs. Winter outcropping
locations of the lighter surface are in the subtropics and the denser surface
in the subpolar regions (Fig. 1). Depth locates
the neutral surface and its gradients give a sense of vertical geostrophic shear.
Acceleration potential contours are streamlines of geostrophic velocity on that
surface relative to 900 dbar. Salinity on a neutral surface is a passive tracer
inversely related to temperature since saltier water must be cooler and fresher
water must be warmer to remain on the neutral surface. Finally, the planetary
component of the potential vorticity is a dynamically active tracer that (away
from the equator and the western boundary) has seldom been described in the
Tropics, but see Gouriou
and Toole (1993) . In the absence of mixing and boundary forcing,
both salinity and potential vorticity are conservative on neutral surfaces.
However, in the ocean, these properties are not strictly conserved. Their distribution
on neutral surfaces is governed by both advection and diffusion.
, is chosen to lie below the
pycnocline, but within the equatorial pycnostad and the SCCs. Winter outcropping
locations of the lighter surface are in the subtropics and the denser surface
in the subpolar regions (Fig. 1). Depth locates
the neutral surface and its gradients give a sense of vertical geostrophic shear.
Acceleration potential contours are streamlines of geostrophic velocity on that
surface relative to 900 dbar. Salinity on a neutral surface is a passive tracer
inversely related to temperature since saltier water must be cooler and fresher
water must be warmer to remain on the neutral surface. Finally, the planetary
component of the potential vorticity is a dynamically active tracer that (away
from the equator and the western boundary) has seldom been described in the
Tropics, but see Gouriou
and Toole (1993) . In the absence of mixing and boundary forcing,
both salinity and potential vorticity are conservative on neutral surfaces.
However, in the ocean, these properties are not strictly conserved. Their distribution
on neutral surfaces is governed by both advection and diffusion.
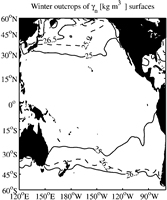
Figure 1. Winter (Jan–Mar in the north and Jul–Sep in the south)
surface outcrops of  n =
25.0 and 26.5 kg m
n =
25.0 and 26.5 kg m and the pycnocline
base (dashed lines) from seasonal climatologies of temperature (Levitus
and Boyer 1994) and salinity (Levitus
et al. 1994).
and the pycnocline
base (dashed lines) from seasonal climatologies of temperature (Levitus
and Boyer 1994) and salinity (Levitus
et al. 1994).
The  n = 25.0 kg m
n = 25.0 kg m surface has a specific volume anomaly of 301 (± 2) × 10
surface has a specific volume anomaly of 301 (± 2) × 10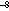 m
m kg
kg and a potential density anomaly of 24.984 (± 0.004) kg m
and a potential density anomaly of 24.984 (± 0.004) kg m ,
very close to the second lightest surface of Tsuchiya
(1968). The Northern and Southern Hemisphere patterns differ largely.
Depth (Fig. 2a) shoals from over 225 m in the
northwest part of the region to less than 25 m off South America. A nearly zonal
ridge starts at 7°N off the western boundary, reaches 11°N by 125°W,
and terminates at its shallowest in the Costa Rica Dome (somewhat obscured in
this large-scale climatology) at 9°N, 90°W (Hoffman
et al. 1981). This ridge lies below the upwelling-favorable wind
stress associated with the ITCZ and separates the NEC and the NECC. A nearly
zonal trough starts at 3°N off the western boundary and reaches 5°N
by 105°W. East of 150°W, this trough separates the NECC to the north
from the SEC to the south. A ridge along the equator marks the dome associated
with the upper part of the EUC and separates the northern and southern branches
of the SEC. At the southern edge a marked eastward shoaling occurs about 120°W,
with isobaths fanning out equatorward and westward, a signature of the SEC.
At least some of the shoaling just off South America is owing to upwelling-favorable
alongshore winds there.
,
very close to the second lightest surface of Tsuchiya
(1968). The Northern and Southern Hemisphere patterns differ largely.
Depth (Fig. 2a) shoals from over 225 m in the
northwest part of the region to less than 25 m off South America. A nearly zonal
ridge starts at 7°N off the western boundary, reaches 11°N by 125°W,
and terminates at its shallowest in the Costa Rica Dome (somewhat obscured in
this large-scale climatology) at 9°N, 90°W (Hoffman
et al. 1981). This ridge lies below the upwelling-favorable wind
stress associated with the ITCZ and separates the NEC and the NECC. A nearly
zonal trough starts at 3°N off the western boundary and reaches 5°N
by 105°W. East of 150°W, this trough separates the NECC to the north
from the SEC to the south. A ridge along the equator marks the dome associated
with the upper part of the EUC and separates the northern and southern branches
of the SEC. At the southern edge a marked eastward shoaling occurs about 120°W,
with isobaths fanning out equatorward and westward, a signature of the SEC.
At least some of the shoaling just off South America is owing to upwelling-favorable
alongshore winds there.
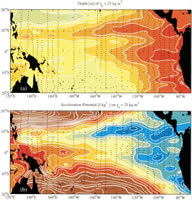
Figure 2a. Maps of properties on  n
= 25.0 kg m
n
= 25.0 kg m , within the tropical pycnocline.
Color palettes are consistent for Figs. 2–5 except for acceleration potential,
which has a varying offset. The locations of the mean hydrographic profiles
(black dots) are given by the average positions of the CTD stations within bins
mentioned in section 2. All panels are objectively mapped from values at these
locations. (a) Depth, contour intervals of 25 m. (b) Acceleration potential
relative to 900 dbar, contour intervals of 0.25 J kg
, within the tropical pycnocline.
Color palettes are consistent for Figs. 2–5 except for acceleration potential,
which has a varying offset. The locations of the mean hydrographic profiles
(black dots) are given by the average positions of the CTD stations within bins
mentioned in section 2. All panels are objectively mapped from values at these
locations. (a) Depth, contour intervals of 25 m. (b) Acceleration potential
relative to 900 dbar, contour intervals of 0.25 J kg .
.
Acceleration potential on this surface (Fig. 2b)
has a similar pattern to that of depth, but reversed in sign. A trough extends
from 6°N off the western boundary to 11°N, 125°W and terminates
around 9°N, 90°W. The eastward fall of values along this trough from
165°E to 135°W reveals interior flow southward from the NEC into the
NECC. Most of these isopleths can be traced northeast, originating off North
America. While the eastern part of this southward flow may be part of a tropical
recirculation centered at 11°N, 125°W, this pattern is weaker on lighter
neutral surfaces (not shown). A ridge on the equator with values falling between
the dateline and 105°W suggests equatorward pycnocline convergence feeding
the EUC. In the north, between the dateline and 150°W the NECC supplies
this convergence from the western boundary. From 150° to 100°W a ridge
at 3°–4°N separates the NECC from the SEC. Values falling eastward
along this ridge again indicate some southward interior flow from the NECC into
the SEC and subsequent convergence on the equator to feed the EUC. These southward
flows from the NEC to NECC starting at 165°E extending at most to 135°W
and from the NECC to the SEC from 150° to 100°W allow a somewhat convoluted
interior route for subtropical water from the northeast Pacific to reach the
EUC.
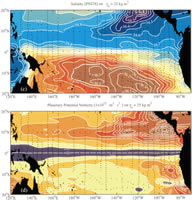
Figure 2b. (c) Salinity, contour intervals of 0.1 (PSS-78) . (d) Planetary
potential vorticity, contour intervals of 200 × 10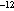 m
m s
s .
.
The Southern Hemisphere is simpler, lacking an ITCZ except in the west. Values
of acceleration potential (Fig. 2b) generally
fall equatorward and eastward to a trough at 3°–2°S from the date
line to about 100°W. This trough separates the SEC from the EUC. The falling
values all along this trough suggest a direct interior route for subtropical
water to reach the EUC from the southeast Pacific. However, west of the date
line a trough is evident at 6°S in the vicinity of the South Pacific convergence
zone, blocking the interior route to the equator. Eastward flow north of this
trough may be related to the South Equatorial Countercurrent (SECC). However,
this surface is too dense to fully reveal the SECC. In the west the SEC feeds
the New Guinea Coastal Undercurrent (Tsuchiya
et al. 1989).
Salinity on this surface (Fig. 2c) is relatively
high in the north-central Pacific, owing to a shallow salinity maximum of the
North Pacific tropical water (NPTW; Tsuchiya
et al. 1989) at  n
= 24.3 kg m
n
= 24.3 kg m . The NPTW is swept westward
and slightly southward in the NEC. The southeast Pacific is very salty, owing
to the strong South Pacific tropical water (SPTW) salinity maximum at
. The NPTW is swept westward
and slightly southward in the NEC. The southeast Pacific is very salty, owing
to the strong South Pacific tropical water (SPTW) salinity maximum at  n
= 24.6 kg m
n
= 24.6 kg m . The SPTW is swept westward
and equatorward in the SEC. In the northeast Pacific, fresher California Current
water (CCW; Tsuchiya
1968), with a salinity minimum at
. The SPTW is swept westward
and equatorward in the SEC. In the northeast Pacific, fresher California Current
water (CCW; Tsuchiya
1968), with a salinity minimum at  n
= 24.8 kg m
n
= 24.8 kg m , sweeps southward and westward
in the NEC, forming the relative minimum on this surface that separates the
NPTW and SPTW. In addition, the denser salinity minimum at
, sweeps southward and westward
in the NEC, forming the relative minimum on this surface that separates the
NPTW and SPTW. In addition, the denser salinity minimum at  n
= 26.7 kg m
n
= 26.7 kg m , a signature of the North
Pacific Intermediate Water (NPIW), may exert some influence, even at this shallow
surface, through diapycnal fluxes into the strong shallow pycnocline under the
ITCZ. Finally, freshness from excess rainfall under the ITCZ may also mix down
to this surface. The equatorial convergence (Fig.
2b) of these three fresh northern sources and the salty SPTW creates a strong
meridional salinity gradient at the equator. Slight northward penetration of
SPTW across the equator is evident near the western boundary. In addition, the
meridional salinity maximum at 1°S between 135° and 100°W is
owing to SPTW advected eastward in the EUC.
, a signature of the North
Pacific Intermediate Water (NPIW), may exert some influence, even at this shallow
surface, through diapycnal fluxes into the strong shallow pycnocline under the
ITCZ. Finally, freshness from excess rainfall under the ITCZ may also mix down
to this surface. The equatorial convergence (Fig.
2b) of these three fresh northern sources and the salty SPTW creates a strong
meridional salinity gradient at the equator. Slight northward penetration of
SPTW across the equator is evident near the western boundary. In addition, the
meridional salinity maximum at 1°S between 135° and 100°W is
owing to SPTW advected eastward in the EUC.
The planetary component of potential vorticity on this surface (Fig.
2d) also exhibits contrasting patterns in the Northern and Southern Hemispheres.
At the northern edge low potential vorticity, especially from 160°W to
125°W, is the remnant of eastern STMW (discussed below) formed to the north.
Under the ITCZ values peak at 10°N, 125°W and again at 11°N,
95°W. This peak under the ITCZ is where the pycnocline is drawn closest
to the surface by Ekman pumping associated with the large-scale wind stress
curl and strengthened, perhaps by some combination of mixing and surface buoyancy
flux. The pattern there is striking because acceleration potential contours
(Fig. 2b) parallel isovorts (Fig.
2d), showing how the southward flow from the NEC to the NECC does not cross
the high potential vorticity under the ITCZ, but moves around it. In the Southern
Hemisphere, where the sign of potential vorticity is reversed, the only high
magnitudes are off South America, where the pycnocline shoals and strengthens.
Elsewhere, potential vorticity is low and relatively uniform. This pattern is
associated with eastern STMW that has a vertical minimum around  n
= 25.3 kg m
n
= 25.3 kg m , visible in vertical sections.
This minimum is strongest at the southern boundary around 120°W where the
surface shoals rapidly from west to east (Fig. 2a).
The uniformity of potential vorticity in the Southern Hemisphere shows there
is no impediment to direct interior convergence of water from the subtropics
to the equator in the SEC. Finally, isovorts around the equator are spread in
the west and pinched in the east, a pattern consistent with acceleration potential
and salinity distributions that suggest equatorward interior pycnocline convergence
feeding the EUC.
, visible in vertical sections.
This minimum is strongest at the southern boundary around 120°W where the
surface shoals rapidly from west to east (Fig. 2a).
The uniformity of potential vorticity in the Southern Hemisphere shows there
is no impediment to direct interior convergence of water from the subtropics
to the equator in the SEC. Finally, isovorts around the equator are spread in
the west and pinched in the east, a pattern consistent with acceleration potential
and salinity distributions that suggest equatorward interior pycnocline convergence
feeding the EUC.
The  n = 26.5 kg m
n = 26.5 kg m surface has a specific volume anomaly of 165 (±2) × 10
surface has a specific volume anomaly of 165 (±2) × 10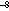 m
m kg
kg and a potential density anomaly of 26.438 (±0.006) kg m
and a potential density anomaly of 26.438 (±0.006) kg m ,
close to the densest surface described by Tsuchiya
(1968). The fields for this surface are markedly different from those
within the pycnocline. Poleward and westward the depth of this surface (Fig.
3a) exceeds 500 m in the NEC and 425 m in the SEC. A ridge under the ITCZ
starts at 6°N off the western boundary, peaks at less than 150 m at 10°N,
135°W, and extends eastward to 7°N, 100°W. In the Southern Hemisphere,
a similar ridge starts at 3°S off the western boundary and turns at 150°W
to reach 15°S off South America. It is shallowest near 9°S, 95°W.
An equatorial trough, from bowing at the base of the EUC, deepens toward both
boundaries.
,
close to the densest surface described by Tsuchiya
(1968). The fields for this surface are markedly different from those
within the pycnocline. Poleward and westward the depth of this surface (Fig.
3a) exceeds 500 m in the NEC and 425 m in the SEC. A ridge under the ITCZ
starts at 6°N off the western boundary, peaks at less than 150 m at 10°N,
135°W, and extends eastward to 7°N, 100°W. In the Southern Hemisphere,
a similar ridge starts at 3°S off the western boundary and turns at 150°W
to reach 15°S off South America. It is shallowest near 9°S, 95°W.
An equatorial trough, from bowing at the base of the EUC, deepens toward both
boundaries.
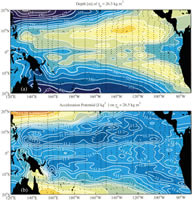
Figure 3a. Maps of properties on  n
= 26.5 kg m
n
= 26.5 kg m , below the tropical pycnocline.
Details follow Fig. 2. (a) Depth, contour intervals of 25 m. (b) Acceleration
potential relative to 900 dbar, contour intervals varying from 0.1 to 0.25 J
kg
, below the tropical pycnocline.
Details follow Fig. 2. (a) Depth, contour intervals of 25 m. (b) Acceleration
potential relative to 900 dbar, contour intervals varying from 0.1 to 0.25 J
kg .
.
Acceleration potential (Fig. 3b) again has
some inverse correlation with depth. A nearly zonal trough starts at 5°N
off the western boundary and reaches 6°N by 100°W. This trough delineates
a cyclonic gyre with a narrow, rapid equatorward limb (the NSCC) and a wider,
slower poleward limb starting at 20°N, 120°W and reaching 10°N
by the western boundary (the deep NEC). These limbs are clearly connected by
southward flow in the west and northward flow in the east. In the south, an
analogous, but more tenuous gyre defined by the 11.5 J kg contour starts at 5°S in the west and reaches 15°S just off South
America. The SSCC forms the equatorward limb and the deep SEC the poleward limb.
The poleward flow connecting these currents in the east probably occurs so close
to the continent that it is not resolved by the climatology, although diapycnal
mixing may also play a role in the mass balance near the eastern boundary. Acceleration
potential does not reveal flow at very low latitudes, but direct velocity measurements
there suggest some westward return flow equatorward of the SCCs, implying deep
anticyclonic gyres (Rowe
et al. 2000). Notably, all these gyres effectively isolate the equator
from deep interior subtropical ventilation.
contour starts at 5°S in the west and reaches 15°S just off South
America. The SSCC forms the equatorward limb and the deep SEC the poleward limb.
The poleward flow connecting these currents in the east probably occurs so close
to the continent that it is not resolved by the climatology, although diapycnal
mixing may also play a role in the mass balance near the eastern boundary. Acceleration
potential does not reveal flow at very low latitudes, but direct velocity measurements
there suggest some westward return flow equatorward of the SCCs, implying deep
anticyclonic gyres (Rowe
et al. 2000). Notably, all these gyres effectively isolate the equator
from deep interior subtropical ventilation.
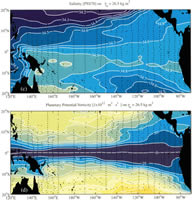
Figure 3b. (c) Salinity, contour intervals of 0.1 (PSS-78). (d) Planetary
potential vorticity, contour intervals of 25 × 10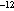 m
m s
s .
.
This surface is fresh at the northern edge, owing to the combined influence
of lighter CCW to the east and denser NPIW to the west (Fig.
3c). In the southeast the fresh influence of Antarctic Intermediate Water
(AAIW), a vertical salinity minimum at  n
= 27.2 kg m
n
= 27.2 kg m , is evident. Higher Coral
Sea and equatorial values encompassed by the 34.8 isohaline are owing to the
mixing of salt from above. The meridional maximum starting at 4°S off the
western boundary and reaching 7°S off South America is consistent with
eastward advection in the SSCC. The salinity front at 2°N off the western
boundary, reaching 4.5°N by 105°W (Fig.
3c), suggests that there is little meridional flow across the NSCC (Gouriou
and Toole 1993). Similarly, another front starting at 10°N off
the western boundary and reaching 20°N by 110°W suggests that meridional
flow is also small at the northern limb of the deep gyre. The relatively homogenous
region between these fronts, with slightly fresher subtropical waters advected
eastward in the equatorward limb of the gyre and slightly saltier equatorial
waters advected westward in the northern limb, is consistent with a tracer swirling
around the gyre edge and homogenizing in the gyre interior.
, is evident. Higher Coral
Sea and equatorial values encompassed by the 34.8 isohaline are owing to the
mixing of salt from above. The meridional maximum starting at 4°S off the
western boundary and reaching 7°S off South America is consistent with
eastward advection in the SSCC. The salinity front at 2°N off the western
boundary, reaching 4.5°N by 105°W (Fig.
3c), suggests that there is little meridional flow across the NSCC (Gouriou
and Toole 1993). Similarly, another front starting at 10°N off
the western boundary and reaching 20°N by 110°W suggests that meridional
flow is also small at the northern limb of the deep gyre. The relatively homogenous
region between these fronts, with slightly fresher subtropical waters advected
eastward in the equatorward limb of the gyre and slightly saltier equatorial
waters advected westward in the northern limb, is consistent with a tracer swirling
around the gyre edge and homogenizing in the gyre interior.
Potential vorticity on this surface (Fig. 3d)
increases nearly monotonically in magnitude poleward. However, lower values
in the east are associated with the thickening equatorial pycnostad. In the
Southern Hemisphere, a strong meridional front separating the equatorial pycnostad
from the subtropical waters is evident, starting at 3°S off the western
boundary and reaching 15°S off South America. This front runs along the
SSCC, suggesting an absence of meridional exchange across this current. In the
Northern Hemisphere, a similar front starts at 2°N off the western boundary
and extends to about 5°N, 105°W. As in the salinity field, there is
another front associated with the northern limb of this deep cyclonic gyre starting
at 20°N, 110°W and reaching 10°N off the western boundary. Also
paralleling the salinity field, between these fronts there is a relatively homogenous
region where high subtropical values are advected eastward in the NSCC and low
tropical values are swept northward in the eastern Pacific and then westward
in the deep NEC. The low values under the ITCZ and off the South American coast
suggest that upwelling-driven vortex stretching below the pycnocline is associated
with the poleward transport in the deep cyclonic gyres.
In summary, property maps on two neutral surfaces highlight differences between
the two hemispheres within the tropical pycnocline as well as changes at the
pycnocline base. The pycnocline shoals from west to east, and under the ITCZ.
In the north, equatorward flow of relatively fresh pycnocline water follows
a serpentine route to skirt westward of the eastern potential vorticity maximum
under the ITCZ. In the south, relatively salty pycnocline water follows a much
more direct interior route to the equator through a region of homogenous and
low potential vorticity characteristic of strong eastern STMW there. Below the
pycnocline, deep cyclonic gyres are evident in each hemisphere, precluding any
interior equatorward flow there. Acceleration potential, salinity, and potential
vorticity distributions are consistent with advection around these deep gyres,
and homogenization within them, especially in the northern hemisphere.
4. Meridional-vertical water property sections
To complement the maps on neutral surfaces, two sets of meridional-vertical
sections are compared and contrasted, one along 165°E, representative of
the western Pacific, and another along 125°W, representative of the eastern
Pacific. These sections are similar in areal extent to a set of mean sections
used to discuss currents and water masses in the central Pacific (Wyrtki
and Kilonsky 1984). Sections of salinity and planetary potential
vorticity are displayed with neutral surfaces (referred to as isopycnals) overlaid
and are discussed as tracers of the net meridional circulation and the deep
cyclonic gyres.
The latitude ranges, maximum densities and pressures, and core densities and
pressures of the quasi-zonal tropical currents (Table 1) generally shoal with
the pycnocline, tilted upward to the east by the trade winds and shift poleward
from west to east. The eastward-flowing Subtropical Countercurrent (STCC), associated
with northward isopycnal spreading in the STMW, and is only present at 165°E
where the STMW is strong. The NEC at 125°W is poleward of its location
at 165°E where its northern end flows beneath the STCC. The NECC at 125°W
is also poleward and shallower than it is at 165°E. The maximum extents
and cores of the SCCs are poleward, shallower, and lighter at 125°W than
at 165°E. Unlike at 165°E, these currents are easily distinguished
from the EUC and the NECC at 125°W. The EUC is shallower and lighter at
125°W than at 165°E. The north branch of the SEC at 125°W is
shallower, lighter and extends farther north than at 165°E. The south branch
of the SEC at 125°W is shallower, lighter, and stronger than at 165°E.
The SECC is only evident at 165°E. These zonal shifts imply geostrophic
equatorward convergence within the pycnocline and divergence below it.
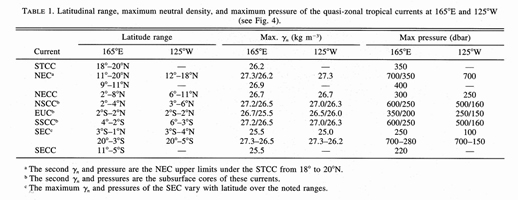
Table 1. Latitudinal range, maximum neutral density, and maximum pressure
of the quasi-zonal tropical currents at 165°E and 125°W (see Fig.
4).
At 165°E (Fig. 4a), the NPTW salinity maximum
at  n = 24.0 kg m
n = 24.0 kg m near 20°N is reduced, partly owing to zonal flow, under the ITCZ around
8°N between the fresh surface waters and the NPIW. Here the NPIW salinity
minimum near
near 20°N is reduced, partly owing to zonal flow, under the ITCZ around
8°N between the fresh surface waters and the NPIW. Here the NPIW salinity
minimum near  n = 26.8 kg
m
n = 26.8 kg
m at 20°N dominates the fresh northern
water approaching the equator along serpentine routes, lightening to
at 20°N dominates the fresh northern
water approaching the equator along serpentine routes, lightening to  n
= 26.1 kg m
n
= 26.1 kg m by 1°N. This density
shift of the salinity minimum is realized because only the lightest portion
of the NPIW influence flows toward the equator in the interior and that from
the western boundary flows east in the NSCC. In the south, fresh AAIW is noticeable
at depth. The SPTW salinity maximum contrasts strongly with the fresh northern
water as both converge on the EUC. The interruption of the 35.8 isohaline at
10°S is associated with fresh eastward flow in the SECC. Around
by 1°N. This density
shift of the salinity minimum is realized because only the lightest portion
of the NPIW influence flows toward the equator in the interior and that from
the western boundary flows east in the NSCC. In the south, fresh AAIW is noticeable
at depth. The SPTW salinity maximum contrasts strongly with the fresh northern
water as both converge on the EUC. The interruption of the 35.8 isohaline at
10°S is associated with fresh eastward flow in the SECC. Around  n
= 26.8 kg m
n
= 26.8 kg m a southern salty influence
can be seen within the entire deep equatorial pycnostad between 3°S and
3°N. The local meridional minimum at 5°N, the northern edge of the
NSCC, is advected eastward, as are slightly salty waters at 5°S in the
southern edge of the SSCC. Around this same density, a homogenous local vertical
maximum between 5°N to 11°N and 250 to 450 m is the result of mixing
at the center of the deep cyclonic gyre. This gyre separates the fresh waters
to the north from the saltier equatorial waters.
a southern salty influence
can be seen within the entire deep equatorial pycnostad between 3°S and
3°N. The local meridional minimum at 5°N, the northern edge of the
NSCC, is advected eastward, as are slightly salty waters at 5°S in the
southern edge of the SSCC. Around this same density, a homogenous local vertical
maximum between 5°N to 11°N and 250 to 450 m is the result of mixing
at the center of the deep cyclonic gyre. This gyre separates the fresh waters
to the north from the saltier equatorial waters.
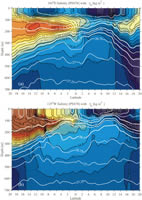
Figure 4. Mean meridional-vertical sections of salinity contoured at 0.1
intervals (PSS-78) with contours of  n
at varying intervals (thick lines 25.0, 26.0, and 26.5 kg m
n
at varying intervals (thick lines 25.0, 26.0, and 26.5 kg m )
overlaid in white. Color palettes follow Fig. 2. Nominal vertical exaggeration
is 5000:1. (a) 165°E. (b) 125°W.
)
overlaid in white. Color palettes follow Fig. 2. Nominal vertical exaggeration
is 5000:1. (a) 165°E. (b) 125°W.
At 125°W (Fig. 4b) the salinity minimum
is shallower and lighter than at 165°E; CCW influence at  n
= 25.5 kg m
n
= 25.5 kg m near 20°N persists as
a subtle vertical minimum to 1°N at
near 20°N persists as
a subtle vertical minimum to 1°N at  n
= 25.0 kg m
n
= 25.0 kg m , roughly following neutral
surfaces on its serpentine equatorward path. Where the pycnocline is shallowest,
around 12°N, the fresh surface influence of precipitation under the ITCZ
may augment that of the CCW. The northern deep salinity minimum is much weaker
and denser than at 165°E, reflecting an absence of NPIW influence here.
The AAIW salinity minimum around
, roughly following neutral
surfaces on its serpentine equatorward path. Where the pycnocline is shallowest,
around 12°N, the fresh surface influence of precipitation under the ITCZ
may augment that of the CCW. The northern deep salinity minimum is much weaker
and denser than at 165°E, reflecting an absence of NPIW influence here.
The AAIW salinity minimum around  n
= 27.2 kg m
n
= 27.2 kg m at 20°S is stronger than
at 165°E, being closer to the southeast Pacific where AAIW is freshest.
Above the AAIW is the strong SPTW salinity maximum at
at 20°S is stronger than
at 165°E, being closer to the southeast Pacific where AAIW is freshest.
Above the AAIW is the strong SPTW salinity maximum at  n
= 24.5 kg m
n
= 24.5 kg m . This maximum is formed by
excess evaporation over precipitation where the neutral surface outcrops in
the southeast Pacific, which is why it is stronger here that at 165°E.
An isolated vertical maximum at 1°S,
. This maximum is formed by
excess evaporation over precipitation where the neutral surface outcrops in
the southeast Pacific, which is why it is stronger here that at 165°E.
An isolated vertical maximum at 1°S,  n
= 25.0 kg m
n
= 25.0 kg m (not visible in Fig.
4b, but see Fig. 2c) is SPTW advected eastward
in the EUC, where convergence creates a strong contrast with fresher northern
water. Around
(not visible in Fig.
4b, but see Fig. 2c) is SPTW advected eastward
in the EUC, where convergence creates a strong contrast with fresher northern
water. Around  n = 26.5
kg m
n = 26.5
kg m , salty southern influence is present
within the entire deep equatorial pycnostad, now between 5°S and 5°N.
Again local meridional extrema are advected eastward at the poleward edges of
the SCCs, now 6° from the equator. Around this same density, a homogenous
local vertical maximum between 7°N and 13°N is the result of mixing
within the center of the northern deep cyclonic gyre. All these deep equatorial
influences are poleward of and shallower than their locations at 165°E,
consistent with potential vorticity conservation below eastward pycnocline shoaling.
In addition, these influences are about 0.3 kg m
, salty southern influence is present
within the entire deep equatorial pycnostad, now between 5°S and 5°N.
Again local meridional extrema are advected eastward at the poleward edges of
the SCCs, now 6° from the equator. Around this same density, a homogenous
local vertical maximum between 7°N and 13°N is the result of mixing
within the center of the northern deep cyclonic gyre. All these deep equatorial
influences are poleward of and shallower than their locations at 165°E,
consistent with potential vorticity conservation below eastward pycnocline shoaling.
In addition, these influences are about 0.3 kg m lighter at 125°W than at 165°E, suggestive of diapycnal mixing.
lighter at 125°W than at 165°E, suggestive of diapycnal mixing.
At 165°E, the pycnocline is strongest at  n
= 23.5 kg m
n
= 23.5 kg m , and potential vorticity there
(Fig. 5a) is of roughly equal magnitude in both
hemispheres. STMW is evident at 20°N as a weak minimum at
, and potential vorticity there
(Fig. 5a) is of roughly equal magnitude in both
hemispheres. STMW is evident at 20°N as a weak minimum at  n
= 25.5 kg m
n
= 25.5 kg m , around the base of the STCC.
In the south, there is no corresponding vertical minimum, as 165°E is far
from the Southern Hemisphere eastern STMW source. Around
, around the base of the STCC.
In the south, there is no corresponding vertical minimum, as 165°E is far
from the Southern Hemisphere eastern STMW source. Around  n
= 26.7 kg m
n
= 26.7 kg m , the equatorial pycnostad
is manifest as a subtle local vertical minimum from about 3°S to 3°N
(not visible in Fig. 5a). Slightly denser, around
, the equatorial pycnostad
is manifest as a subtle local vertical minimum from about 3°S to 3°N
(not visible in Fig. 5a). Slightly denser, around
 n = 27.0 kg m
n = 27.0 kg m ,
vertical minima expand poleward to 5°S (barely visible) and 11°N,
marking the equatorial influence in the deep cyclonic gyres poleward of the
SCCs. As in the property maps (Fig. 3), the
southern deep cyclonic gyre is more subtle than the northern deep cyclonic gyre.
Within these deep gyres below the pycnocline, the orthogonality of nearly vertical
isovorts and nearly horizontal isopycnals suggest an absence of significant
adiabatic meridional flows.
,
vertical minima expand poleward to 5°S (barely visible) and 11°N,
marking the equatorial influence in the deep cyclonic gyres poleward of the
SCCs. As in the property maps (Fig. 3), the
southern deep cyclonic gyre is more subtle than the northern deep cyclonic gyre.
Within these deep gyres below the pycnocline, the orthogonality of nearly vertical
isovorts and nearly horizontal isopycnals suggest an absence of significant
adiabatic meridional flows.
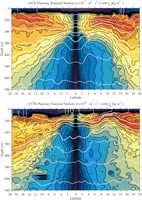
Figure 5. Mean meridional-vertical sections of planetary potential vorticity
contoured at logarithmic intervals (10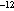 m
m s
s )
with contours of
)
with contours of  n at varying
intervals (thick lines 25.0, 26.0, and 26.5 kg m
n at varying
intervals (thick lines 25.0, 26.0, and 26.5 kg m )
overlaid in white. Color palettes follow Fig. 2. Nominal vertical exaggeration
is 5000:1. (a) 165°E. (b) 125°W.
)
overlaid in white. Color palettes follow Fig. 2. Nominal vertical exaggeration
is 5000:1. (a) 165°E. (b) 125°W.
At 125°W, potential vorticity (Fig. 5b)
is highest in magnitude within the pycnocline, around  n
= 24.0 kg m
n
= 24.0 kg m , slightly denser but shallower
than at 165°E, probably because the mixed layer is lighter and deeper in
the west. Potential vorticity is high under the ITCZ from 9°N to 13°N,
where the pycnocline is closest to the surface. This maximum, much higher than
at 165°E, precludes direct meridional flow. As shown above (Fig.
3), water within the pycnocline must circumnavigate the high potential vorticity
under the ITCZ on its way from the subtropics to the equator. At 20°N,
the local vertical minimum at
, slightly denser but shallower
than at 165°E, probably because the mixed layer is lighter and deeper in
the west. Potential vorticity is high under the ITCZ from 9°N to 13°N,
where the pycnocline is closest to the surface. This maximum, much higher than
at 165°E, precludes direct meridional flow. As shown above (Fig.
3), water within the pycnocline must circumnavigate the high potential vorticity
under the ITCZ on its way from the subtropics to the equator. At 20°N,
the local vertical minimum at  n
= 24.5 kg m
n
= 24.5 kg m is a signature of eastern
STMW that underlies the NPTW salinity maximum. In the Southern Hemisphere eastern
STMW is much more clearly evident as a broad vertical minimum between
is a signature of eastern
STMW that underlies the NPTW salinity maximum. In the Southern Hemisphere eastern
STMW is much more clearly evident as a broad vertical minimum between  n
= 25.0 kg m
n
= 25.0 kg m and
and  n
= 26.0 kg m
n
= 26.0 kg m . The meridional homogeneity
in Southern Hemisphere eastern STMW is the result of stratification increasing
linearly towards the equator to compensate the shrinking Coriolis parameter
and conserve potential vorticity. Hence in contrast to the northern pycnocline,
there is no impediment to direct meridional flow toward the equator within the
southern pycnocline. Around
. The meridional homogeneity
in Southern Hemisphere eastern STMW is the result of stratification increasing
linearly towards the equator to compensate the shrinking Coriolis parameter
and conserve potential vorticity. Hence in contrast to the northern pycnocline,
there is no impediment to direct meridional flow toward the equator within the
southern pycnocline. Around  n
= 26.5 kg m
n
= 26.5 kg m , the deep equatorial pycnostad
is evident in the local vertical minimum from 5°S to 5°N. Slightly
denser, around
, the deep equatorial pycnostad
is evident in the local vertical minimum from 5°S to 5°N. Slightly
denser, around  n = 26.8
kg m
n = 26.8
kg m , vertical minima expand poleward
to 10°S (barely visible) and 13°N, marking the equatorial influence
in the deep cyclonic gyres poleward of the SCCs. As in the salinity field, these
deep equatorial influences are all poleward of, lighter than, and shallower
than analogous features at 165°E. The poleward spread of low potential
vorticity below the pycnocline from west to east suggests deep interior meridional
flow is poleward in the presence of eastward zonal flow.
, vertical minima expand poleward
to 10°S (barely visible) and 13°N, marking the equatorial influence
in the deep cyclonic gyres poleward of the SCCs. As in the salinity field, these
deep equatorial influences are all poleward of, lighter than, and shallower
than analogous features at 165°E. The poleward spread of low potential
vorticity below the pycnocline from west to east suggests deep interior meridional
flow is poleward in the presence of eastward zonal flow.
In summary, these two sections show the shoaling and strengthening of the pycnocline
from west to east. Poleward shifts of the NEC and NECC from west to east are
apparent, as are shoalings and poleward shifts of the SCCs. The salinity sections
show the contrast at the equator between the strong salty SPTW in the southeast
and the combined fresh northern influence of CCW in the northeast, surface water
under the ITCZ, and NPIW in the northwest. Lateral extrema within the SCCs and
the eastern EUC are suggestive of eastward zonal advection. The deeper homogeneity
in salinity between the deep SEC and the SSCC, as well as between the deep NEC
and the NSCC, suggests mixing within deep cyclonic gyres bounded by these currents.
These gyres are associated with a lack of interior meridional convergence toward
the equator across the Pacific in both hemispheres. The potential vorticity
sections highlight the contrast between the Southern and Northern Hemisphere
pycnoclines, with the ridge of high potential vorticity under the ITCZ presenting
an obstacle to direct meridional flow in the northeast, but smaller barriers
in the west and no such impediment in the southeast. STMWs are evident as vertical
minima in potential vorticity under salinity maxima in the northwest and especially
in the southeast. In addition, the low potential vorticity and high salinity
within the deep cyclonic gyres in each hemisphere is consistent with a lack
of equatorward convergence below the pycnocline. In fact, poleward spreading
of these equatorial influences to the east between the SSCC and the deep SEC
and between the deep NEC and the NSCC supports arguments for a deep poleward
interior flow.
5. Interior quasi-meridional geostrophic mass transport estimates
Geostrophic calculations are made relative to a reference surface of 900 dbar,
roughly  n = 27.5 kg m
n = 27.5 kg m .
Inspection of the depth of this isopycnal (not shown) suggests that geostrophic
shear at this surface is weak except in the far southwest portion of the region
under investigation, where the subtropical pycnocline is deep. This assumption
is supported by direct measurements at this level (Davis
1998). The 900-dbar surface sits well beneath the tropical pycnocline
and its use as a reference surface captures all the geostrophic shear above.
Three previous quantitative works on zonal mass transports across the Tropics
used similar surfaces: 600 dbar at 165°E (Gouriou
and Toole 1993), 1000 dbar between 150°W and 158°W (Wyrtki
and Kilonsky 1984), and 500 dbar at 110°W (Hayes
et al. 1983).
.
Inspection of the depth of this isopycnal (not shown) suggests that geostrophic
shear at this surface is weak except in the far southwest portion of the region
under investigation, where the subtropical pycnocline is deep. This assumption
is supported by direct measurements at this level (Davis
1998). The 900-dbar surface sits well beneath the tropical pycnocline
and its use as a reference surface captures all the geostrophic shear above.
Three previous quantitative works on zonal mass transports across the Tropics
used similar surfaces: 600 dbar at 165°E (Gouriou
and Toole 1993), 1000 dbar between 150°W and 158°W (Wyrtki
and Kilonsky 1984), and 500 dbar at 110°W (Hayes
et al. 1983).
Quasi-meridional interior geostrophic mass transport estimates presented here
are made in a layer extending from the base of the mixed layer to the base of
the tropical pycnocline. The results are similar to those inferred independently
from wind fields using Sverdrup dynamics (McPhaden
and Fine 1988), but here the magnitudes of the interior meridional
transports are quantified as well as the density range over which they occur.
The mixed layer is excluded because the mean wind field over nearly the entire
region dictates a poleward Ekman transport (Lu
and McCreary 1995), which overwhelms the equatorward geostrophic
velocity in the mixed layer. Our functional definition of the tropical pycnocline
base in this instance is the neutral density at which the net interior meridional
mass transport changes sign from equatorward above to poleward below within
a 9° latitude band containing the deep cyclonic gyres in each hemisphere.
In the Northern Hemisphere, from 3° to 11°N, the base sits at  n
= 25.9 ± 0.2 kg m
n
= 25.9 ± 0.2 kg m . In the southern
hemisphere, from 4 to 12°S, the base sits at
. In the southern
hemisphere, from 4 to 12°S, the base sits at  n
= 26.2 ± 0.2 kg m
n
= 26.2 ± 0.2 kg m (see Fig.
1 for isopycnal winter outcrop locations). Interior meridional transports
at 8°N and 8°S show convergent equatorward flow, hence interior communication
from the subtropics to the equator above these isopycnals and divergent poleward
flow in the deep cyclonic gyres below them (Fig.
6). Only interior transports are presented here. While western boundary
currents in both hemispheres transport significant amounts of subtropical pycnocline
water toward the equator, they have been extensively discussed elsewhere (Tsuchiya
et al. 1989; Lukas
et al. 1991; Butt
and Lindstrom 1994; Wijffels
et al. 1995).
(see Fig.
1 for isopycnal winter outcrop locations). Interior meridional transports
at 8°N and 8°S show convergent equatorward flow, hence interior communication
from the subtropics to the equator above these isopycnals and divergent poleward
flow in the deep cyclonic gyres below them (Fig.
6). Only interior transports are presented here. While western boundary
currents in both hemispheres transport significant amounts of subtropical pycnocline
water toward the equator, they have been extensively discussed elsewhere (Tsuchiya
et al. 1989; Lukas
et al. 1991; Butt
and Lindstrom 1994; Wijffels
et al. 1995).

Figure 6. Net meridional mass transport (10 kg s
kg s ) excluding the mixed layer binned
in
) excluding the mixed layer binned
in  n every 0.2 kg m
n every 0.2 kg m from the South American coast to (a) 165°E at 8°S and (b) 135°E
at 8°N. In the south, equatorward flow is concentrated near the eastern
STMW density and poleward flow below
from the South American coast to (a) 165°E at 8°S and (b) 135°E
at 8°N. In the south, equatorward flow is concentrated near the eastern
STMW density and poleward flow below  n
= 26.1 kg m
n
= 26.1 kg m . In the north, equatorward
flow is at lighter densities and poleward flow below
. In the north, equatorward
flow is at lighter densities and poleward flow below  n
= 25.9 kg m
n
= 25.9 kg m . (c) Downward accumulated
integrals of (a) and (b) show the net interior transport within the pycnocline
(to
. (c) Downward accumulated
integrals of (a) and (b) show the net interior transport within the pycnocline
(to  n roughly 26 kg m
n roughly 26 kg m )
in the northern hemisphere (6 × 10
)
in the northern hemisphere (6 × 10 kg
s
kg
s ) is much less than that in the southern
hemisphere (14 × 10
) is much less than that in the southern
hemisphere (14 × 10 kg s
kg s ).
).
In the Southern Hemisphere, meridional mass transport estimates within the
pycnocline, from  n = 26.2
kg m
n = 26.2
kg m to the base of the mixed layer, are
made from 17°S equatorward. South of 17°S data starts too far off
the South American coast for a meaningful interior meridional transport estimate.
At all latitudes the interior meridional transport ends well east of the western
boundary, and equatorward of 14°S this transport starts west of South America.
The interior transport occurs between 135°W and South America at 17°S,
but shifts westward toward the equator and is found between 170°W and 110°W
by 6°S. Between 17°S and 6°S the estimates have a minimum of
13 (± 5) × 10
to the base of the mixed layer, are
made from 17°S equatorward. South of 17°S data starts too far off
the South American coast for a meaningful interior meridional transport estimate.
At all latitudes the interior meridional transport ends well east of the western
boundary, and equatorward of 14°S this transport starts west of South America.
The interior transport occurs between 135°W and South America at 17°S,
but shifts westward toward the equator and is found between 170°W and 110°W
by 6°S. Between 17°S and 6°S the estimates have a minimum of
13 (± 5) × 10 kg s
kg s at 9°S and a maximum of 19 (± 2) × 10
at 9°S and a maximum of 19 (± 2) × 10 kg s
kg s at 16°S, both latitudes where
data are sparse and error estimates are large. From 17°S to 6°S the
weighted average and standard deviation of interior meridional mass transport
is 15 (± 1) × 10
at 16°S, both latitudes where
data are sparse and error estimates are large. From 17°S to 6°S the
weighted average and standard deviation of interior meridional mass transport
is 15 (± 1) × 10 kg s
kg s (Fig. 7a). This value is an estimate of the interior
subtropical mass transport from the Southern Hemisphere toward the equator.
For purposes of comparison, this interior meridional mass transport estimate
slightly exceeds the 9–12 (× 10
(Fig. 7a). This value is an estimate of the interior
subtropical mass transport from the Southern Hemisphere toward the equator.
For purposes of comparison, this interior meridional mass transport estimate
slightly exceeds the 9–12 (× 10 m
m s
s )
of southern origin estimated to join the EUC core from western boundary currents
in the Southern Hemisphere (Butt
and Lindstrom 1994). These results are in reasonable accord with
analysis of a data assimilation product which suggest western boundary and interior
transports at 10°S of 15 and 11 (× 10
)
of southern origin estimated to join the EUC core from western boundary currents
in the Southern Hemisphere (Butt
and Lindstrom 1994). These results are in reasonable accord with
analysis of a data assimilation product which suggest western boundary and interior
transports at 10°S of 15 and 11 (× 10 m
m s
s ),
respectively (Huang
and Liu 1999).
),
respectively (Huang
and Liu 1999).

Figure 7. Quasi-meridional interior pycnocline (mixed-layer base to pycnocline
base) mass transport (10 kg s
kg s )
zonally accumulated from the Americas westward. Symbols are individual integration
points and lines are objectively mapped assuming a Gaussian covariance with
a correlation length scale of 20° longitude and an error-to-signal energy
of 0.25. (a) Southern hemisphere transports at three latitudes (17°S solid
line, 12°S dashed, and 7°S dot-dashed) with a pycnocline base at
)
zonally accumulated from the Americas westward. Symbols are individual integration
points and lines are objectively mapped assuming a Gaussian covariance with
a correlation length scale of 20° longitude and an error-to-signal energy
of 0.25. (a) Southern hemisphere transports at three latitudes (17°S solid
line, 12°S dashed, and 7°S dot-dashed) with a pycnocline base at  n
= 26.2 kg m
n
= 26.2 kg m . (b) Northern hemisphere transport
between the NEC and NECC with a pycnocline base at
. (b) Northern hemisphere transport
between the NEC and NECC with a pycnocline base at  n
= 25.9 kg m
n
= 25.9 kg m . (c) Northern hemisphere transport
between the NECC and SEC with a pycnocline base at
. (c) Northern hemisphere transport
between the NECC and SEC with a pycnocline base at  n
= 25.9 kg m
n
= 25.9 kg m .
.
Equatorward of 6°S, meridional interior transport estimates increase from
20 (± 2) × 10 kg s
kg s between 170°W and 110°W at 5°S to 30 (± 4) × 10
between 170°W and 110°W at 5°S to 30 (± 4) × 10 kg s
kg s between 170°W and 120°W
at 3°S. While low-latitude geostrophic calculations are noisy, the trend
is clear. This rapid increase probably reflects the presence of a shallow meridional
overturning cell at the equator, with interior equatorward geostrophic transport
working to offset large poleward mass transports in the Ekman layer. This cell
has been observed in profiling and acoustic Doppler current meter data from
the Hawaii-Tahiti Shuttle Experiment (McPhaden
1984; Johnson
and Luther 1994) and reproduced in numerical models (Lu
et al. 1998).
between 170°W and 120°W
at 3°S. While low-latitude geostrophic calculations are noisy, the trend
is clear. This rapid increase probably reflects the presence of a shallow meridional
overturning cell at the equator, with interior equatorward geostrophic transport
working to offset large poleward mass transports in the Ekman layer. This cell
has been observed in profiling and acoustic Doppler current meter data from
the Hawaii-Tahiti Shuttle Experiment (McPhaden
1984; Johnson
and Luther 1994) and reproduced in numerical models (Lu
et al. 1998).
The Northern Hemisphere is somewhat more complicated than the Southern Hemisphere,
with the quasi-zonal NEC, NECC, SEC, and EUC alternating in flow direction.
Instead of presenting simple meridional mass transports, which would contain
portions of these quasi-zonal currents, we isolate the cross-gyre transports
by calculating transports across the geopotential anomaly trough under the ITCZ
that separates the NEC from the NECC (Fig. 7b).
Another estimate is made across the lower-latitude ridge that separates the
NECC from the SEC and 2°N at a few western longitudes where no ridge is
evident (Fig. 7c). In both cases, there are regions
of little net transport east and west of where the interior meridional transport
occurs. Within the pycnocline, from  n
= 25.9 kg m
n
= 25.9 kg m to the base of the mixed layer,
–5 (± 1) × 10
to the base of the mixed layer,
–5 (± 1) × 10 kg s
kg s flows across the trough from the NEC to the NECC between 165°E and 135°W.
This value is an estimate of the interior subtropical mass transport from the
Northern Hemisphere toward the equator, and is one third the estimate of 15
(± 1) × 10
flows across the trough from the NEC to the NECC between 165°E and 135°W.
This value is an estimate of the interior subtropical mass transport from the
Northern Hemisphere toward the equator, and is one third the estimate of 15
(± 1) × 10 kg s
kg s equatorward interior flow estimated for the Southern Hemisphere. A simple comparison
of interior pycnocline meridional mass transports along 8°N and 8°S
gives a similar result (Fig. 6c). The Northern
Hemisphere interior transport estimate is probably an upper bound because it
may contain southward flow from a tropical recirculation around the ITCZ centered
near 11°N, 125°W (Fig. 2b). However,
since there is no evidence of any significant northward component of the recirculation
east of 125°W (Fig. 7b), the net tropical
recirculation is likely to be small. For purposes of comparison, this northern
interior transport is less than half of the 13–16 (× 10
equatorward interior flow estimated for the Southern Hemisphere. A simple comparison
of interior pycnocline meridional mass transports along 8°N and 8°S
gives a similar result (Fig. 6c). The Northern
Hemisphere interior transport estimate is probably an upper bound because it
may contain southward flow from a tropical recirculation around the ITCZ centered
near 11°N, 125°W (Fig. 2b). However,
since there is no evidence of any significant northward component of the recirculation
east of 125°W (Fig. 7b), the net tropical
recirculation is likely to be small. For purposes of comparison, this northern
interior transport is less than half of the 13–16 (× 10 m
m s
s )
of northern origin water estimated to reside within the core of the EUC well
to the west at 153°E (Butt
and Lindstrom 1994). These results are again in reasonable accord
with analysis of a data assimilation product, which suggests western boundary
and interior transports at 10°N of 14 and 3 × 10
)
of northern origin water estimated to reside within the core of the EUC well
to the west at 153°E (Butt
and Lindstrom 1994). These results are again in reasonable accord
with analysis of a data assimilation product, which suggests western boundary
and interior transports at 10°N of 14 and 3 × 10 m
m s
s ,
respectively (Huang
and Liu 1999).
,
respectively (Huang
and Liu 1999).
Farther to the south, an estimated –35 (± 8) × 10 kg s
kg s flows across the low-latitude ridge
from the NECC to the SEC between 165°E and 105°W (Fig.
7c). The 30 (± 8) × 10
flows across the low-latitude ridge
from the NECC to the SEC between 165°E and 105°W (Fig.
7c). The 30 (± 8) × 10 kg s
kg s increase in equatorward transport with relation to the northern estimate seems
large and may be an upper bound, since part of the transport calculation is
made at 2°N, a fairly low latitude at which to apply geostrophy. However,
this increase can fairly easily be accounted for by a combination of two similarly
sized sources. First, the addition of northern subtropical water from the Mindanao
Current (Lukas
et al. 1991; Wijffels
et al. 1995) at the western boundary transported eastward within
the NECC could be contributing to equatorward pycnocline mass flux. The NECC
carries 20 × 10
increase in equatorward transport with relation to the northern estimate seems
large and may be an upper bound, since part of the transport calculation is
made at 2°N, a fairly low latitude at which to apply geostrophy. However,
this increase can fairly easily be accounted for by a combination of two similarly
sized sources. First, the addition of northern subtropical water from the Mindanao
Current (Lukas
et al. 1991; Wijffels
et al. 1995) at the western boundary transported eastward within
the NECC could be contributing to equatorward pycnocline mass flux. The NECC
carries 20 × 10 m
m s
s at 165°E (Gouriou and Toole 1993)
and only 8 × 10
at 165°E (Gouriou and Toole 1993)
and only 8 × 10 m
m s
s by 110°W (Hayes et al. 1983), allowing
for an increase of 12 × 10
by 110°W (Hayes et al. 1983), allowing
for an increase of 12 × 10 m
m s
s in equatorward transport from the NECC
to the SEC. Second, the poleward Ekman transport across the low-latitude ridge
is 45 × 10
in equatorward transport from the NECC
to the SEC. Second, the poleward Ekman transport across the low-latitude ridge
is 45 × 10 kg s
kg s between 165°E and 105°W using the Hellerman
and Rosenstein (1983) surface wind stress climatology after applying
a recommended scaling factor of 0.8 to correct the drag coefficient (Harrison
1989). If 40 (± 25)% of the equatorward geostrophic transport
balancing this Ekman transport occurs in a shallow tropical meridional cell
extending below the mixed layer (Lu
et al. 1998), it would account for the remaining mass budget within
interior meridional transport errors.
between 165°E and 105°W using the Hellerman
and Rosenstein (1983) surface wind stress climatology after applying
a recommended scaling factor of 0.8 to correct the drag coefficient (Harrison
1989). If 40 (± 25)% of the equatorward geostrophic transport
balancing this Ekman transport occurs in a shallow tropical meridional cell
extending below the mixed layer (Lu
et al. 1998), it would account for the remaining mass budget within
interior meridional transport errors.
6. Discussion
The focus of this manuscript is on describing interior pycnocline pathways
from the subtropics to the equator in the Pacific Ocean and quantifying mass
fluxes of these pathways. The serpentine pathway in the Northern Hemisphere
has been described using the potential vorticity and salinity fields, as well
as quantified with geostrophic calculations. Relatively fresh water flows westward
in the NEC, skirting the high potential vorticity under the ITCZ, then flows
south from the NEC to the NECC between 165°E and 135°W, then eastward
in the NECC, then south between 150°W and 100°W to join the SEC and
flow westward and converge on the EUC. The more direct interior pathway in the
Southern Hemisphere has also been studied, with salty low potential vorticity
water flowing northward and westward. At 17°S this flow occurs between
135°W and the South American coast and by 6°S between 170°W and
110°W, continuing northward and westward to the equator, augmented by a
shallow meridional overturning cell.
The Southern Hemisphere interior route carries about three times more subtropical
water towards the equator than does the northern interior route. At least two,
probably related, factors contribute to this difference. First, a large body
of modeling work reviewed in the introduction as well as the data analysis presented
here has demonstrated that high potential vorticity under the ITCZ is obviously
linked to the relatively small equatorward interior flow found in the north.
Most equatorward flow in the Northern Hemisphere pycnocline flows around this
region of high potential vorticity to the western boundary where it can more
easily turn southward. Second, there is evidence for a hemispheric asymmetry
in eastern STMW production rates and locations. The Northern Hemisphere region
of high subduction rate associated with eastern STMW production is centered
near 25°N, 130°W (Huang
and Qiu 1994; Hautala
and Roemmich 1998). The analogous southern region is centered near
20°S, 120°W (Huang
and Qiu 1998). The area of large subduction rate associated with
eastern STMW production in the south is nearly twice the magnitude and twice
the area of that in the north. In addition the southern region is located eastward
and equatorward of the northern region. All of these differences contribute
to a stronger equatorward interior flux of eastern STMW in the Southern Hemisphere.
The low potential vorticity evident in the pycnocline of the Southeast Pacific
(Figs. 2d and 5b)
as well as the large equatorward transport of water around the density of eastern
STMW in the south compared to that in the north (Fig.
6) reflect this asymmetry in production rates and locations.
The other significant result of this manuscript is that the salinity, potential
vorticity, and geostrophic velocity fields are all consistent with deep cyclonic
gyres below the pycnocline, hence an absence of interior equatorward flow of
subtropical water there. The poleward interior flows of these gyres are in opposition
to the equatorward flows within the pycnocline, the base of which we defined
to be near the 26 kg m neutral surface.
The eastward flowing SCCs serve as the equatorward limbs of these deep cyclonic
gyres and the deeper portions of the westward flowing NEC and SEC serve as their
poleward limbs. Salinity and potential vorticity signals are advected around
these gyres, with reduced meridional gradients in the gyre interiors.
neutral surface.
The eastward flowing SCCs serve as the equatorward limbs of these deep cyclonic
gyres and the deeper portions of the westward flowing NEC and SEC serve as their
poleward limbs. Salinity and potential vorticity signals are advected around
these gyres, with reduced meridional gradients in the gyre interiors.
The dynamics of these deep gyres are not clear. In addition to the cyclonic
gyres poleward of the SCCs there is evidence for anticyclonic gyres equatorward
of the SCCs (Rowe
et al. 2000). The eastward zonal flows within the SCCs have been
modeled as inertial jets (Johnson
and Moore 1997). The westward quasi-zonal return flows poleward of
the SCCs are part of the deep NEC and SEC, but are not well studied. More return
flow equatorward of the SCCs may be in the Equatorial Intermediate Current (Rowe
et al. 2000). Flows completing the gyres at the western boundaries
presumably follow western boundary current dynamics. Examination of acceleration
potential and potential vorticity (Figs. 3b
and 3d) suggests that in both deep cyclonic
gyres contours of these quantities cross in the western boundary, where dissipation
is expected. Upwelling under the ITCZ and off the South American coast may force
the poleward flow within these deep cyclonic gyres through vortex stretching
and diapycnal processes. In the southern deep gyre the effects of upwelling
are likely confined to the coast, and are not well resolved with this data set.
However, in the northern deep gyre contours cross again in the region of poleward
flow under the ITCZ, where vortex stretching and diapycnal processes are posited.
The eastward lightening of the equatorial influences in these deep gyres discussed
here, as well as the change in density of the SCCs (Johnson
and Moore 1997) is consistent with the hypothesized influence diapycnal
processes.
Acknowledgments. This work was stimulated by conversations with E. Firing,
W. Kessler, J. McCreary, D. Rowe, and, as always, L. Thompson. E. Firing, W.
Kessler, J. McCreary, and an anonymous review all helped to improve the manuscript.
K. McTaggart helped with calibration and processing of CTD data. This work was
funded by the NOAA Office of Oceanic and Atmospheric Research, the NOAA Office
of Global Programs, and the NASA Physical Oceanography Program.
References
Akima, H., 1970: A new method of interpolation and smooth curve fitting based
on local procedures. J. Assoc. Comput. Mach., 17, 589–603.
Butt, J., and E. Lindstrom, 1994: Currents of the east coast of New Ireland,
Papua New Guinea, and their relevance to the regional undercurrents in the Western
Equatorial Pacific Ocean. J. Geophys. Res., 99, 12,503–12,514.
Davis, R. E., 1998: Preliminary results from directly measuring middepth circulation
in the tropical and South Pacific. J. Geophys. Res., 103, 24,619–24,639.
Deser, C., M. A. Alexander, and M. S. Timlin, 1996: Upper-ocean thermal variations
in the North Pacific during 1970–1991. J. Climate, 9, 1840–1855.
Fine, R. A., W. H. Peterson, and H. G. Ostlund, 1987: The penetration of tritium
into the tropical Pacific. J. Phys. Oceanogr., 17, 553–564.
Gouriou, Y., and J. Toole, 1993: Mean circulation of the upper layers of the
western equatorial Pacific Ocean. J. Geophys. Res., 98, 22,495–22,520.
Gu, D., and G. S. Philander, 1997: Interdecadal climate fluctuations that depend
on exchanges between the tropics and extratropics. Science, 275,
805–807.
Harrison, D. E., 1989: On climatological monthly mean wind stress and wind
stress curl fields over the World Ocean. J. Climate, 2, 57–70.
Hautala, S. L., and D. H. Roemmich, 1998: Subtropical mode water in the Northeast
Pacific Basin. J. Geophys. Res., 103, 13,055–13,066.
Hayes, S. P., J. M. Toole, and L. J. Mangum, 1983: Water-mass and transport
variability at 110°W in the Equatorial Pacific. J. Phys. Oceanogr.,
13, 153–168.
Hellerman, S., and M. Rosenstein, 1983: Normal monthly wind stress over the
world ocean with error estimates. J. Phys. Oceanogr., 13, 1093–1104.
Hoffman, E. E., A. J. Busalacchi, and J. J. O'Brien, 1981: Wind generation
of the Costa Rica Dome. Science, 214, 552–554.
Huang, B., and Z. Liu, 1999: Pacific subtropical-tropical thermocline water
exchange in the National Centers for Environmental Prediction ocean model. J.
Geophys. Res., 104, 11,065–11,076.
Huang, R. X., and B. Qiu, 1994: Three-dimensional structure of the wind-driven
circulation in the subtropical North Pacific. J. Phys. Oceanogr., 24,
1608–1622.
Huang, R. X., and B. Qiu, 1998: The structure of the wind-driven circulation
in the subtropical South Pacific Ocean. J. Phys. Oceanogr., 28,
1173–1186.
Jackett, D., and T. J. McDougall, 1997: A neutral density variable for the
world's ocean. J. Phys. Oceanogr., 27, 237–263.
Johnson, E. S., and D. S. Luther, 1994: Mean zonal momentum balance in the
upper and central equatorial Pacific Ocean. J. Geophys. Res., 99,
7689–7705.
Johnson, G. C., and D. W. Moore, 1997: The Pacific subsurface countercurrents
and an inertial model. J. Phys. Oceanogr., 27, 2448–2459.
Levitus, S., and T. P. Boyer, 1994: World Ocean Atlas 1994, Vol. 4: Temperature:
NOAA Atlas NESDIS 4, U.S. Govt. Printing Office, 117 pp.
Levitus, S., R. Burgett, and T. P. Boyer, 1994: World Ocean Atlas 1994,
Vol. 4: Salinity: NOAA Atlas NESDIS 3, U.S. Govt. Printing Office, 99 pp.
Liu, Z., 1994: A simple model of the mass exchange between the subtropical
and tropical ocean. J. Phys. Oceanogr., 24, 1153–1165.
Liu, Z., and B. Huang, 1998: Why is there a tritium maximum in the central
equatorial Pacific thermocline? J. Phys. Oceanogr., 28, 1527–1533.
Liu, Z., S. G. H. Philander, and R. C. Pacanowski, 1994: A GCM study of the
tropical-subtropical upper-ocean water exchange. J. Phys. Oceanogr.,
24, 2606–2623.
Lu, P., and J. P. McCreary Jr., 1995: Influence of the ITCZ on the flow of
thermocline water from the subtropical to the equatorial Pacific Ocean. J.
Phys. Oceanogr., 25, 3076–3088.
Lu, P., J. P. McCreary Jr., and B. A. Klinger, 1998: Meridional circulation
cells and the source waters of the Pacific equatorial undercurrent. J. Phys.
Oceanogr., 28, 62–84.
Lukas, R., E. Firing, P. Hacker, P. L. Richardson, C. A. Collins, R. Fine,
and R. Gammon, 1991: Observations of the Mindanao Current during the Western
Equatorial Pacific Ocean Circulation Study. J. Geophys. Res., 96,
7089–7104.
Luyten, J. R., J. Pedlosky, and H. Stommel, 1983: The ventilated thermocline.
J. Phys. Oceanogr., 13, 292–309.
Lysne, J., P. Chang, and B. Giese, 1997: Impact of the extratropical Pacific
on equatorial variability. Geophys. Res. Lett., 24, 2589–2592.
McCreary, J. P., and P. Lu, 1994: On the interaction between the subtropical
and the equatorial oceans: The subtropical cell. J. Phys. Oceanogr.,
24, 466–497.
McPhaden, M. J., 1984: On the dynamics of the equatorial subsurface countercurrents.
J. Phys. Oceanogr., 14, 1216–1225.
McPhaden, M. J., and R. A. Fine, 1988: A dynamical interpretation of the tritium
maximum in the Central Equatorial Pacific. J. Phys. Oceanogr., 18,
1454–1457.
Rothstein, L. M., R.-H. Zhang, A. J. Busalacchi, and D. Chen, 1998: A numerical
simulation of the mean water pathways in the subtropical and tropical Pacific
Ocean. J. Phys. Oceanogr., 28, 322–342.
Rowe, G. D., E. Firing, and G. C. Johnson, 2000: Pacific Equatorial subsurface
countercurrent velocity, transport, and potential vorticity. J. Phys. Oceanogr.,
30, 1172–1187.
Schneider, N., A. J. Miller, M. A. Alexander, and C. Deser, 1999: Subduction
of decadal North Pacific temperature anomalies: Observations and dynamics. J.
Phys. Oceanogr., 29, 1056–1070.
Tsuchiya, M., 1968: Upper waters of the intertropical Pacific Ocean. Johns
Hopkins Oceanogr. Stud., 4, 50 p.
Tsuchiya, M., 1975: Subsurface countercurrents in the eastern equatorial Pacific
Ocean. J. Mar. Res., 33 (Suppl.), 145–175.
Tsuchiya, M., R. Lukas, R. A. Fine, E. Firing, and E. Lindstrom, 1989: Source
waters of the Pacific Equatorial Undercurrent. Progress in Oceanography,
Vol. 23, Pergamon, 101–147.
Wijffels, S., E. Firing, and J. Toole, 1995: The mean structure and variability
of the Mindanao Current at 8°N. J. Geophys. Res., 100, 18,421–18,435.
Wyrtki, K., 1975: Fluctuations of dynamic topography in the Pacific Ocean.
J. Phys. Oceanogr., 5, 450–459.
Wyrtki, K., and B. Kilonsky, 1984: Mean water and current structure during
the Hawaii-to-Tahiti shuttle experiment. J. Phys. Oceanogr., 14,
242–254.
Zhang, R.-H., L. M. Rothstein, and A. J. Busalacchi, 1998: Origin of upper-ocean
warming and El Niño change on decadal scales in the tropical Pacific Ocean.
Nature, 391, 879–883.
Go to Gallery of Figures and Tables
PMEL Outstanding Papers
PMEL Publications Search
PMEL Homepage
 kg s–1. In contrast, the southern branch of the South Equatorial
Current clearly brings pycnocline water estimated at 15 (± 1) × 10
kg s–1. In contrast, the southern branch of the South Equatorial
Current clearly brings pycnocline water estimated at 15 (± 1) × 10 kg s–1 from the southern subtropics directly to the equator
in the South Pacific through an interior region of low and relatively uniform
potential vorticity. In both hemispheres, these interior pathways extend downward
as far as the lightest waters of the equatorial pycnostad. The subsurface countercurrents
flanking the pycnostad form the equatorward limbs of tropical subsurface cyclonic
gyres. These deep gyres are consistent with the absence of interior ventilation
of the equator from the subtropics below the pycnocline. Measured and derived
fields from a hydrographic climatology are presented on neutral surfaces and
in meridional-vertical sections to show that salinity, potential vorticity,
and acceleration potential are all consonant with these arguments. The vertical
extent of interior communication is also in agreement with the transient tracer
results.
kg s–1 from the southern subtropics directly to the equator
in the South Pacific through an interior region of low and relatively uniform
potential vorticity. In both hemispheres, these interior pathways extend downward
as far as the lightest waters of the equatorial pycnostad. The subsurface countercurrents
flanking the pycnostad form the equatorward limbs of tropical subsurface cyclonic
gyres. These deep gyres are consistent with the absence of interior ventilation
of the equator from the subtropics below the pycnocline. Measured and derived
fields from a hydrographic climatology are presented on neutral surfaces and
in meridional-vertical sections to show that salinity, potential vorticity,
and acceleration potential are all consonant with these arguments. The vertical
extent of interior communication is also in agreement with the transient tracer
results. = 23 to 26.2 kg m–3 and that at
= 23 to 26.2 kg m–3 and that at  the timescale for water to
reach the equator is less than 14 years.
the timescale for water to
reach the equator is less than 14 years.
 n, surfaces (
n, surfaces ( , which is calculated
from the mean hydrographic profiles and then smoothed with a 35-dbar median
filter before use. The median filter effectively reduces noise while preserving
the rapid transition from the surface mixed layer to the pycnocline. This quantity
is then used to calculate the planetary component of the potential vorticity,
Q = N
, which is calculated
from the mean hydrographic profiles and then smoothed with a 35-dbar median
filter before use. The median filter effectively reduces noise while preserving
the rapid transition from the surface mixed layer to the pycnocline. This quantity
is then used to calculate the planetary component of the potential vorticity,
Q = N
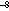 m
m and a potential density anomaly of 24.984 (± 0.004) kg m
and a potential density anomaly of 24.984 (± 0.004) kg m

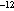 m
m





 m
m s
s