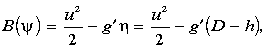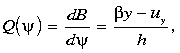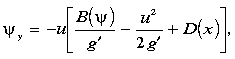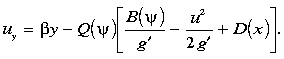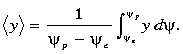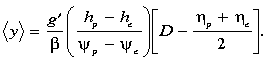U.S. Dept. of Commerce / NOAA / OAR / PMEL
/ Publications
The Pacific Subsurface Countercurrents and an Inertial Model
Gregory C. Johnson and Dennis W. Moore
National Oceanic and Atmospheric Administration/Pacific Marine Environmental
Laboratory, Seattle, Washington
Journal of Physical Oceanography, 27, 2448-2459
Not subject to U.S. copyright. Published in 1997 by the American Meterological Society.
5. An inertial model
The simple model inertial jet presented below reproduces many of the properties
of the Tsuchiya jets. At this point, the Tsuchiya jets have been shown to be
features of the equatorial circulation that extend across much of the Pacific
Ocean. Observational characteristics of the SSCCs that are reproduced by the
model are their constant volume transports, their advection of properties over
long zonal distances, their rapidity and narrowness, their poleward shift from
west to east, the large potential vorticity gradients across them, and the pycnostad
between them which builds from west to east.
The essential properties of the model are illustrated in a schematic (Fig.
4). Since the basic dynamics are the same on either side of the equator,
only the north SSCC will be modeled here in the interest of clarity and brevity.
Near the equator, the pycnocline in the Pacific shoals to the east as a result
of the near-balance in the layer above, between zonal pressure gradient and
wind stress (McPhaden
and Taft 1988). Off the equator, the pycnocline shoals to the east as result
of the near-balance of the wind stress curl and the product of poleward mass
transport and  (Sverdrup
1947). However, the dynamics of the surface layer, here termed layer 0,
have been studied extensively elsewhere. Only the observed eastward shoaling
of the pycnocline is vital to the evolution of the model jet, found in layer
1, so the pycnocline depth D, which is the interface between layer
0 and layer 1, is simply set to shoal eastward. The abyss, here termed layer
2, is assumed to be quiescent, allowing reduced-gravity dynamics to hold in
layer 1. Since layer 1 has a positive thickness, h, the depth of the
interface between layers 1 and 2 is
(Sverdrup
1947). However, the dynamics of the surface layer, here termed layer 0,
have been studied extensively elsewhere. Only the observed eastward shoaling
of the pycnocline is vital to the evolution of the model jet, found in layer
1, so the pycnocline depth D, which is the interface between layer
0 and layer 1, is simply set to shoal eastward. The abyss, here termed layer
2, is assumed to be quiescent, allowing reduced-gravity dynamics to hold in
layer 1. Since layer 1 has a positive thickness, h, the depth of the
interface between layers 1 and 2 is  = D - h.
Here D and
= D - h.
Here D and  are negative relative
to the ocean surface. With the focus on layer 1, between the prescribed pycnocline
and the quiescent abyss, the dynamics reduce to a nonlinear model with 1-1/2
layers. The model works with any specified D(x) that is a function
of the zonal direction, x, with no meridional, y, dependence.
are negative relative
to the ocean surface. With the focus on layer 1, between the prescribed pycnocline
and the quiescent abyss, the dynamics reduce to a nonlinear model with 1-1/2
layers. The model works with any specified D(x) that is a function
of the zonal direction, x, with no meridional, y, dependence.
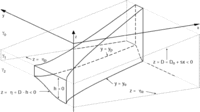
Fig. 4. Perspective view of the model
jet from 30°W of south at 30° elevation (solid lines for visible jet boundaries,
dashed lines for hidden boundaries). The surface layer is not discussed. The
pycnocline depth, D, is the interface between layer 0 and layer 1.
This interface is constant in latitude but slopes linearly up to the east (upper
plane of dotted lines). Since layer 0 is ignored, D can be thought
of as inverted topography at the top of layer 1. The interface depth between
the active layer 1 and the quiescent abyssal layer 2 is  .
This deeper interface is constant on either side of the jet (lower sets of dotted
lines), but slopes up within it, where the velocity, u, is finite.
The interface depths D and
.
This deeper interface is constant on either side of the jet (lower sets of dotted
lines), but slopes up within it, where the velocity, u, is finite.
The interface depths D and  are both negative values referenced to the surface, but their difference, the
layer 1 thickness, h, is a positive quantity. The reduced gravity,
g', at
are both negative values referenced to the surface, but their difference, the
layer 1 thickness, h, is a positive quantity. The reduced gravity,
g', at  is related to the neutral density anomalies,
is related to the neutral density anomalies,  n,
as discussed in section 5. As the pycnocline shoals to the east, the jet edges
ye and yp shift poleward as the jet
thickens, conserving Bernoulli function and potential vorticity on streamlines.
Vertical-meridional exaggeration is 167,000:1 and meridional-zonal exaggeration
is 124:1.
n,
as discussed in section 5. As the pycnocline shoals to the east, the jet edges
ye and yp shift poleward as the jet
thickens, conserving Bernoulli function and potential vorticity on streamlines.
Vertical-meridional exaggeration is 167,000:1 and meridional-zonal exaggeration
is 124:1.
The inviscid momentum equations for the active layer 1 are
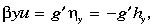 |
(1) |
 |
(2) |
These equations are formulated assuming u »  ,
so that the meridional momentum balance is purely geostrophic but the zonal
momentum balance retains the inertial terms. The reduced gravity g' =
2g(
,
so that the meridional momentum balance is purely geostrophic but the zonal
momentum balance retains the inertial terms. The reduced gravity g' =
2g( 2 -
2 -  1)/(
1)/( 2 +
2 +  1)
is determined by the density difference between the quiescent abyssal layer
2 and the active layer 1 (Fig. 4), where
1)
is determined by the density difference between the quiescent abyssal layer
2 and the active layer 1 (Fig. 4), where
 =
=  n + 1000 kg m-3.
The continuity equation is
n + 1000 kg m-3.
The continuity equation is
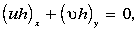 |
(3) |
so a transport streamfunction,  (x,y),
can be defined as
(x,y),
can be defined as
 |
(4a, 4b) |
satisfying (3). From (1) and (2) the Bernoulli function,
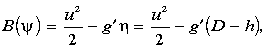 |
(5) |
which includes the zonal kinetic energy as a result of retaining
inertial terms in (2), is conserved following fluid parcels, and therefore must
be a function of  alone. Furthermore, the potential vorticity,
alone. Furthermore, the potential vorticity,
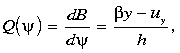 |
(6) |
being the derivative of the Bernoulli function with respect to streamfunction,
is also conserved on streamlines. The potential vorticity includes relative
vorticity from meridional variations in zonal velocity as a result of retaining
inertial terms in (2),
The model boundary conditions are set as follows. At the western edge of the
model domain, longitude x = x0 (140°E),
an inflow profile uo(y) is specified in the active layer,
bounded by quiescent fluid at both the equatorward, ye,
and poleward, yp, edges. Specifying an equatorward interface
depth,  e,
at y = ye and integrating (1) with respect to
y gives the corresponding layer interface depth,
e,
at y = ye and integrating (1) with respect to
y gives the corresponding layer interface depth,  0(y).
After integrating (4a) to find
0(y).
After integrating (4a) to find  at x = x0, (5) and (6) are used to find
B(
at x = x0, (5) and (6) are used to find
B( ) and
Q(
) and
Q( ). It
follows from (1) that, since the fluid is quiescent outside the model jet, the
interface depths at its edges,
). It
follows from (1) that, since the fluid is quiescent outside the model jet, the
interface depths at its edges,  e
and
e
and  p,
are both constants. The jet edges are streamlines,
p,
are both constants. The jet edges are streamlines,  e
and
e
and  p,
so applying (5) shows that zonal speeds at the edges, ue
and up, are also constants.
p,
so applying (5) shows that zonal speeds at the edges, ue
and up, are also constants.
Equations (4a), (5), and (6) can be recast as a set of coupled first-order
differential equations:
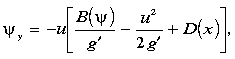 |
(7)
|
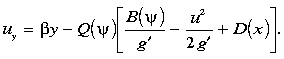 |
(8) |
At each longitude, we guess the location of ye, integrate
(7) and (8) until  =
=  p,
check to see if u = up there, and if not, repeat
the process, adjusting ye until this condition is met. This
solution algorithm has been used before for similar problems (Pedlosky
1987). A monotonic potential vorticity profile across the model jet maintains
sufficient conditions for stability of the basic state (Pedlosky
1979).
p,
check to see if u = up there, and if not, repeat
the process, adjusting ye until this condition is met. This
solution algorithm has been used before for similar problems (Pedlosky
1987). A monotonic potential vorticity profile across the model jet maintains
sufficient conditions for stability of the basic state (Pedlosky
1979).
A number of integrations were carried out, but only one is presented here.
The model pycnocline depth is chosen as D(x) = Do + sx,
and shoals linearly with s = 1.1305 × 10-5
from - 237.5 m at 140°E, the western edge of the model domain,
to -62.5 m at 80°W, the eastern edge of the domain. Here u0(y)
at x0 = 140°E is chosen as a Gaussian with ue = 0.05 m s-1,
up = 0.05 m s-1, a peak velocity
of 0.25 m s-1 at 2.26°N, a decay scale of 0.72° lat, and
edges at 0.09° and 4.61°N. The reduced gravity g' = 5 × 10-3
m s-2, a value characteristic of the density difference between
the abyss and the pycnostad. Setting  e = -375 m
and integrating to yp gives
e = -375 m
and integrating to yp gives  0(y)
and
0(y)
and  p = -300 m.
The net transport is 7.0 × 106 m3 s-1,
close to values of the observed SSCC (Table 1).
p = -300 m.
The net transport is 7.0 × 106 m3 s-1,
close to values of the observed SSCC (Table 1).
For these initial conditions, when (7) and (8) are integrated at x0,
three solutions are found. The existence of multiple solutions should not be
too much of a surprise since the dynamical system is nonlinear and the model
jet edges are free to vary their position. The first solution is the original
Gaussian. A second solution is slightly narrower, with edges shifted poleward
to 0.48° and 4.86°N, a peak velocity of 0.27 m s-1 at 2.20°N,
and an interior velocity minimum near the north edge. The third solution is
the narrowest, a blunted triangular velocity profile extending from 0.68° to
3.77°N with a maximum value of 0.30 m s-1 at 2.25°N (Figs.
4 and 5). The first two solutions get broader,
slower, and quickly develop interior zonal flow minima and then reversals as
the pycnocline shoals to the east. Flow reversals make it impossible to calculate
a solution with the simple procedure outlined here, so we cannot follow these
solutions across the entire domain. The third solution narrows, accelerates,
and does not develop interior flow minima or reversals as the pycnocline shoals
to the east. This third, jetlike solution can be found across the entire domain
and is chosen as the preferable one. E. Firing (1995, personal communication)
has also pointed out that the narrowest, fastest jet has the lowest total energy
for a given B( )
and transport, making it plausibly the most stable flow. This solution does
not match u0(y) and
)
and transport, making it plausibly the most stable flow. This solution does
not match u0(y) and  0(y),
so it is substituted as the initial condition at longitude x0
and carried eastward.
0(y),
so it is substituted as the initial condition at longitude x0
and carried eastward.
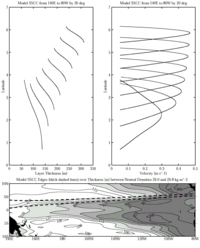
Fig. 5. Active layer thickness within the
model jet plotted against latitude from 140°E to 80°W at 20° intervals (top
left panel) and velocity plotted against latitude from 140°E to 80°W at 20°
intervals (top right panel). Jet edges (thick dashed lines in bottom panel)
are plotted over thickness between  n = 26.0
and 26.8 kg m-3 as in the bottom panel of Fig.
3. The jet shifts poleward, narrows in the meridional, thickens in the vertical,
and accelerates to the east as a consequence of conservation of potential vorticity
and Bernoulli function under the shoaling pycnocline.
n = 26.0
and 26.8 kg m-3 as in the bottom panel of Fig.
3. The jet shifts poleward, narrows in the meridional, thickens in the vertical,
and accelerates to the east as a consequence of conservation of potential vorticity
and Bernoulli function under the shoaling pycnocline.
The solution dynamics are simple. The model is inviscid, so the boundary values
at the western edge of the model jet and the zonal slope of the pycnocline determine
its evolution across the basin. As the jet moves eastward, it is stretched vertically
by the shoaling pycnocline over the quiescent abyss, hence it shifts poleward
to conserve Q. As the jet becomes thicker in the vertical to the east
it accelerates and narrows in the horizontal to conserve transport. Retention
of the inertial terms in (2) allows the jet to narrow and accelerate, making
relative vorticity important in Q conservation near the jet edges.
A totally different behavior is predicted if the model is formulated using the
dynamics of the ventilated thermocline theory (Luyten
et al. 1983), where the geostrophic balance holds for both momentum equations
but the continuity equation remains nonlinear. In this case, the interface depth
alone gives the Bernoulli function and the potential vorticity contains only
the planetary term. Streamlines follow 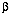 y/h
contours, so they originate on the equator and shift poleward to the east in
direct proportion to the increase in active layer thickness caused by the shoaling
pycnocline. The streamlines under the ventilated thermocline dynamics diverge
to the east whereas those of the chosen inertial solution converge. Hence, the
inclusion of inertial terms, retaining relative vorticity and kinetic energy
in the dynamics, is central to the character of the model jet presented here.
y/h
contours, so they originate on the equator and shift poleward to the east in
direct proportion to the increase in active layer thickness caused by the shoaling
pycnocline. The streamlines under the ventilated thermocline dynamics diverge
to the east whereas those of the chosen inertial solution converge. Hence, the
inclusion of inertial terms, retaining relative vorticity and kinetic energy
in the dynamics, is central to the character of the model jet presented here.
We derive a simple measure of the poleward shift as the model jet moves eastward.
The streamfunction-weighted latitude of the jet, <y>, written
in streamfunction coordinates, is
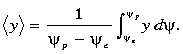 |
(9) |
Some manipulation leads to an expression for <y>
as proportional to the means and differences of various quantities at the jet
edges
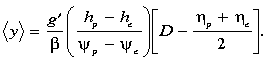 |
(10) |
The term inside the square brackets can also be written as (hp + he)/2.
Thus <y> shifts poleward more quickly if the reduced gravity
is large, the difference in interface depths at the jet edges is large, the
net transport is small, or the pycnocline slope to the east is large.
The model jet shifts poleward just as predicted by the <y> expression
(Figs. 4 and 5).
The model parameters give a <y> that starts at 2.1°N at 140°E
and reaches 5.8°N by 80°W. Mean layer thicknesses within the jet increase steadily
from 106 to 278 m from west to east, a factor of 2.62. The jet narrows
from 3.09° to 0.72° latitude, by a factor of 0.233. The jet accelerates from
a mean velocity of 0.19 to 0.31 m s-1 from west to east,
a factor of 1.63. Multiplication of these factors to obtain unity illustrates
how transport is conserved within the jet. As the jet narrows and thickens,
the thickness increase is offset by a poleward shift in latitude to conserve
potential vorticity overall (Figs. 4 and 5).
The relative vorticity becomes very large at the edges of the jet to conserve
potential vorticity there. At the equatorward edge the tendency of the relative
vorticity is to reduce the magnitude of the potential vorticity while at the
poleward edge the tendency is to increase it. This growth of relative vorticity
near the edges is accomplished through the narrowing of the jet and an increase
in the peak velocity from 0.30 to 0.47 m s-1 from west
to east (Figs. 4 and 5).
Return to previous section or go to next section
PMEL Outstanding Papers
PMEL Publications Search
PMEL Homepage
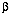 (Sverdrup
1947). However, the dynamics of the surface layer, here termed layer 0,
have been studied extensively elsewhere. Only the observed eastward shoaling
of the pycnocline is vital to the evolution of the model jet, found in layer
1, so the pycnocline depth D, which is the interface between layer
0 and layer 1, is simply set to shoal eastward. The abyss, here termed layer
2, is assumed to be quiescent, allowing reduced-gravity dynamics to hold in
layer 1. Since layer 1 has a positive thickness, h, the depth of the
interface between layers 1 and 2 is
(Sverdrup
1947). However, the dynamics of the surface layer, here termed layer 0,
have been studied extensively elsewhere. Only the observed eastward shoaling
of the pycnocline is vital to the evolution of the model jet, found in layer
1, so the pycnocline depth D, which is the interface between layer
0 and layer 1, is simply set to shoal eastward. The abyss, here termed layer
2, is assumed to be quiescent, allowing reduced-gravity dynamics to hold in
layer 1. Since layer 1 has a positive thickness, h, the depth of the
interface between layers 1 and 2 is  = D - h.
Here D and
= D - h.
Here D and  are negative relative
to the ocean surface. With the focus on layer 1, between the prescribed pycnocline
and the quiescent abyss, the dynamics reduce to a nonlinear model with 1-1/2
layers. The model works with any specified D(x) that is a function
of the zonal direction, x, with no meridional, y, dependence.
are negative relative
to the ocean surface. With the focus on layer 1, between the prescribed pycnocline
and the quiescent abyss, the dynamics reduce to a nonlinear model with 1-1/2
layers. The model works with any specified D(x) that is a function
of the zonal direction, x, with no meridional, y, dependence.
 n,
as discussed in section 5. As the pycnocline shoals to the east, the jet edges
ye and yp shift poleward as the jet
thickens, conserving Bernoulli function and potential vorticity on streamlines.
Vertical-meridional exaggeration is 167,000:1 and meridional-zonal exaggeration
is 124:1.
n,
as discussed in section 5. As the pycnocline shoals to the east, the jet edges
ye and yp shift poleward as the jet
thickens, conserving Bernoulli function and potential vorticity on streamlines.
Vertical-meridional exaggeration is 167,000:1 and meridional-zonal exaggeration
is 124:1. ,
so that the meridional momentum balance is purely geostrophic but the zonal
momentum balance retains the inertial terms. The reduced gravity g' =
2g(
,
so that the meridional momentum balance is purely geostrophic but the zonal
momentum balance retains the inertial terms. The reduced gravity g' =
2g( 2 -
2 -  (x,y),
can be defined as
(x,y),
can be defined as